Using Operations with Scientific Notations
- Operations with Scientific Notation or Standard Form
- Understanding Operations with Scientific Notation
- Multiplying Numbers in Scientific Notation – Steps
- Dividing Numbers in Scientific Notation – Steps
- Adjusting the Solution to Scientific Notation
- Operations with Scientific Notation – Guided Practice
- Operations with Scientific Notation – Real-World Application
- Operations with Scientific Notation – Summary
- Operations with Scientific Notation – Frequently Asked Questions
Learning text on the topic Using Operations with Scientific Notations
Operations with Scientific Notation or Standard Form
In this text, we're going to explore how to perform operations like multiplication and division with numbers expressed in scientific notation. This is also known as standard form. This skill is essential for handling very large or small numbers efficiently in maths and science. We'll also touch on how to convert numbers to scientific notation, providing a comprehensive understanding of this useful mathematical tool.
For a refresher on the scientific notation rules, check out this text: Standard and Scientific Notation.
Remember, Scientific Notation is a way of expressing large or small numbers as a product of a number between 1 and 10 and a power of ten. Standard Notation is our regular way of writing numbers. It is important to know the difference in these key words as sometimes scientific notation is also called standard form which can easily confuse the two representation types of numbers!
Understanding Operations with Scientific Notation
Scientific Notation is a way of expressing numbers that are very large or very small. It's expressed as a product of a number between 1 and 10 and a power of ten and can look like this: $4.3 \times 10^2$. Remember another word for power is exponent.
In the following section, you will learn the steps to finding the product or quotient of numbers in scientific notation.
Multiplying Numbers in Scientific Notation – Steps
- Multiply the decimal parts.
- Add the exponents of 10.
Dividing Numbers in Scientific Notation – Steps
- Divide the decimal parts.
- Subtract the exponent of the divisor from the exponent of the dividend.
Let’s check your understanding so far.
Adjusting the Solution to Scientific Notation
Don’t forget, according to the scientific notation definition, the decimal part should be greater than or equal to 1 and less than 10. Adjusting the decimal and the exponent accordingly will ensure your result is in the correct form of scientific notation.
| Condition | Action | Example Before Adjustment | Example After Adjustment |
|---|---|---|---|
| Decimal is greater than or equal to 10 | Shift decimal left; Increase exponent | $25 × 10^5$ | $2.5 × 10^6$ |
| Decimal is less than 1 | Shift decimal right; Decrease exponent | $0.4 × 10^3 $ | $4.0 × 10^2$ |
Let’s check your understanding so far:
Operations with Scientific Notation – Guided Practice
Let’s walk through some examples of multiplying and dividing numbers in scientific notation:
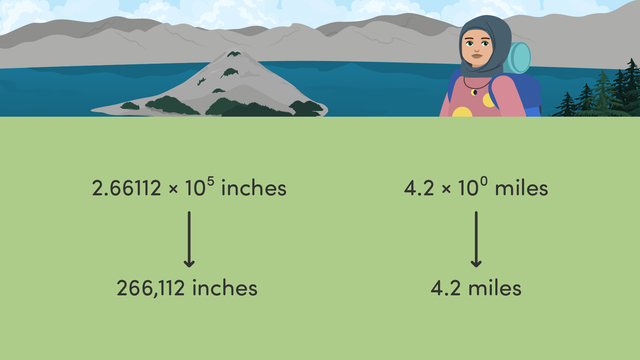
Operations with Scientific Notation – Real-World Application
Now that you have learnt how to multiply and divide numbers written in scientific notation, let’s try to complete a few real-world problems.
Operations with Scientific Notation – Summary
Key Points from This Text:
- Scientific notation simplifies the process of working with very large or small numbers.
- Multiplication involves multiplying the decimal parts and then adding the exponents.
- Division includes dividing the decimal parts and then subtracting the exponents.
- Converting to and from scientific notation is an essential skill in these operations.
Explore more topics and practice problems on our website, including interactive exercises, videos and worksheets to further enhance your understanding of scientific notation and other maths concepts!
If you are looking for more on scientific notation, this video is a great resource: Choosing Appropriate Units with Scientific Notation.
Operations with Scientific Notation – Frequently Asked Questions
Using Operations with Scientific Notations exercise
-
Calculate the standard form multiplication.
HintsTo make it easier rearrange it.
$2\times4$ and $10^{3}\times10^{5}$
When multiplying indices with the same base we ADD the powers.
$2\times4 = 8$
$8\times(10^{3}\times10^{5})$
Finish by putting your answer in standard form.
Solution$8\times10^{8}$
- Multiply $2$ and $4$ together
- Add the powers $3$ and $5$ = $10^{8}$
-
Calculate the standard form division
HintsTo make it easier rearrange it.
$9\div3$ and $10^{7}\div10^{5}$
When dividing indices with the same base we subtract the powers.
$9\div3 = 3$
$3\times(10^{7}\div10^{5})$
Finish by putting your answer in standard form.
Solution$3\times10^{2}$
- Divide $9$ by $3$
- Subtract the powers $7$ and $5$ = $10^{2}$
-
Find the product of two values in standard form
HintsTo make it easier rearrange it.
$4\times6$ and $10^{8}\times10^{4}$
When multiplying indices with the same base we ADD the powers.
$4\times6 = 24$
$24\times(10^{8}\times10^{4}) = 24\times10^{12}$ (We add the powers here)
The answer is not in standard form.
For this $24$ needs to be a number between $1$ and $10$ but this means we have to add one more to the indices.
Solution$2.4\times10^{13}$ in standard form.
-
Calculate and leave the answer in standard form.
HintsSome of the answers will need putting into standard form when the calculation has been done.
For example, if the answer is $27\times10^{3}$, we need to write it as a number between $1$ and $10$ and make adjustments.
$2.7\times10^{4}$
If the answer is already a number between $1$ and $10$ then no adjustment is necessary it is in standard form.
Solution- $(45\times10^{6})\div(3\times10^{2}) = 1.5\times10^{5}$
- $(7\times10^{3})\times(6\times10^{2}) = 4.2\times10^{6}$
- $(6\times10^{4})\times(7\times10^{2}) = 4.2\times10^{7}$
- $(42\times10^{8})\div(6\times10^{2}) = 7\times10^{6}$
-
Multiplying and dividing by powers of ten.
HintsWhen multiplying indices we add the powers if the base is the same.
When dividing indices we subtract the powers if the base is the same.
Solution- $10^{6}\times10^{2} = 10^{8}$
- $10^{3}\times10^{4} = 10^{7}$
- $10^{8}\div10^{2} = 10^{6}$
- $10^{5}\div10^{2} = 10^{3}$
When dividing indices we subtract the powers if the base is the same.
-
Divide the standard form numbers.
HintsThe operation we use for this problem is division.
Distance from London to Sydney $\div$ Distance from London to Paris
Distance from London to Sydney $\div$ Distance from London to Paris
$(1.05\times10^{4})\div(2.9\times10^{2})$
When we have divided $(1.05\times10^{4})\div(2.9\times10^{2})$ we need to make an adjustment to get the answer to the nearest whole number.
SolutionAnswer is $36$

Rational Numbers on the Number Line

Subtracting Integers

Solving Problems with Negative Numbers
Multiplying and Dividing Integers

Rational and Irrational Numbers
Subtracting Rational Numbers by adding the Inverse

Analysing Numerical Patterns
Standard and Scientific Notation
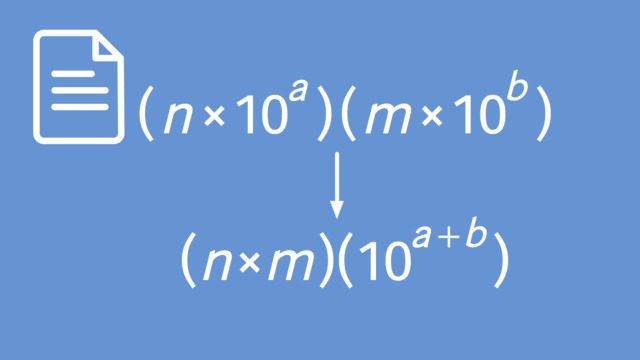
Using Operations with Scientific Notations
Interpreting Scientific Notation
 Do you want to learn faster and more easily?
Do you want to learn faster and more easily?







