Adding Fractions on a Number Line
Basics on the topic Adding Fractions on a Number Line
Adding Fractions on a Number Line
When we learn how to add fractions, we can use visual methods to understand the process better. In this learning text we are going to use number lines as a visual explanation to get a better understanding.
Steps to Adding Fractions on a Number Line
We know that a fraction has a top number (numerator) and a bottom number (denominator). Adding fractions is different from adding whole numbers. Let’s look at the steps to take if you want to add two fractions on a number line.
- Firstly, check if the fractions have common or like denominators.
- Secondly, divide the number line (between 0 and 1) into equal parts and label each part; the denominator is the indicator in how many parts the number line will be divided between whole numbers. Then look at the first fraction and circle or highlight it on the number line.
- Finally jump from the highlighted fraction to the right as many times as shown by the numerator of the second fraction. Remember to simplify your answer if possible!
Adding Fractions on a Number Line – Examples
Let’s look at the examples below and follow the process of adding two fractions on a number line. We are adding here two fractions with a common denominator of eight: $\frac{1}{8}$ and $\frac{5}{8}$. In order to add two fractions using the number line, we divide the number line between zero and one into equal parts and label each part. Then we must find the first fraction on our number line and then jump to the right as many times as the numerator of the fraction we are adding shows. Our added fraction has a numerator of five, so we must jump five times forward. We have landed on $\frac{6}{8}$, so $\frac{1}{8}$ add $\frac{5}{8}$ is $\frac{6}{8}$. Now we can simplify our answer if possible. $\frac{6}{8}$ we can simplify to $\frac{3}{4}$ by dividing the numerator and denominator by a common factor which is two.
Let’s look at another example of adding fractions with a numberline.
This time we have $\frac{2}{6}$ and $\frac{3}{6}$. Both fractions share the same denominator, which is six. We are going to repeat the same process as above. We divide the number line between zero and one into equal parts and label each part. Then we must find the first fraction on our number line and then jump to the right as many times as the numerator of the fraction we are adding shows. Our added fraction has a numerator of three, so we must jump three times forward. We have landed on $\frac{5}{6}$, so $\frac{2}{6}$ add $\frac{3}{6}$ is $\frac{5}{6}$. Now we can simplify our answer if possible. This time we cannot simplify further, so our answer is $\frac{5}{6}$.
Adding Fractions on a Number Line - Further Practice
Today we learned about adding fractions using a number line. Let’s look at the steps below for a quick review.
| Step # | What to do |
|---|---|
| 1. | Check that the fractions have the same denominators. |
| 2. | Divide the number line into equal parts between 0 and 1 as shown by the denominators. |
| 3. | Find your first fraction on your number line and circle or highlight it. |
| 4. | Jump forward to the right as many times as shown by the numerator to find the sum. |
| 5. | Simplify your answer if possible |
To test your knowledge on adding and subtracting fractions on a number line, have a look at our practice problems, videos and worksheets.
Frequently Asked Questions on Adding Fractions on a Number Line
Transcript Adding Fractions on a Number Line
"While I fill the tank, keep your eye on the fuel gauge!" "You got it partner, I'll calculate the total fuel we have!" Let's help Tank calculate the total amount of fuel in the submarine by "Adding Fractions on a Number Line." We can use a number line like this to help us when adding fractions. To add fractions on a number line, first, check that the fractions have the same denominator. One-eighth and five-eighths have the same denominator because the number on the bottom part of each fraction is the same. Next, divide the number line into equal parts, using the denominator to determine the number of parts. Eight is the denominator, so make eight equal parts between zero and one and label them like this. Now find one-eighth on the number line which is here. Then identify the numerator of the fraction we are adding which is five. Jump forward five parts from one-eighth. We land on six-eighths. One-eighth plus five-eighths is six-eighths. Finally, simplify the answer if possible. To simplify fractions, find a common factor for the numerator and denominator that both can be divided into. Six-eighths can be simplified by dividing the numerator and denominator by two making the fraction three-quarters. Now we have looked at the steps needed to add fractions on a number line, let's help calculate how much petrol Axel and Tank have in their submarine! The submarine had two-sixths of petrol left in the tank and Axel added three-sixths to the submarine. With the number line ready, what is the first step? First, check that the fractions have the same denominator. Since both fractions have a six as the bottom part of the fraction, we know that they have the same denominator. What is the next step? Divide the number line into equal parts between zero and one as shown by the denominator, which is six, and label each part on the number line. What should we do next? Find the first fraction, two-sixths which is here. How do we find the sum? We identify the numerator of the fraction we are adding which is three and jump forward three parts from two-sixths. The sum of two-sixths plus three-sixths is five-sixths. Can five-sixths be simplified? Five-sixths cannot be simplified as one is the only factor that goes into both five and six. So we leave the answer as five-sixths. While Axel pays for the petrol, let's review! Remember, when adding fractions on a number line: First, check that the fractions have the same denominator. Next, divide the number line into equal parts between whole numbers as shown by the denominator. Then, locate the first fraction on the number line. Finally, jump forward the number of parts as shown by the numerator of the second fraction to find the sum. Remember to simplify the fraction if you can. "Alright, are you ready to hit the road, Tank?" "I was born ready, partner."
Adding Fractions on a Number Line exercise
-
What are the steps to add fractions?
HintsBefore you start adding the fractions, what do you need to check is the same?
Once you have divided your number line into equal parts, what do you need to locate?
SolutionFirst, check that the fractions you are adding have the same denominator.
Next, divide the number line into equal parts as shown by the denominators.
Then, locate the first fraction on the number line.
Finally, count forward the number of parts, as shown by the numerator on the second fraction.
-
How much petrol is in the tank?
HintsEach interval on the number line goes up in steps of $\frac1 6$. How many jumps will be needed to add $\frac3 6$?
There was already $\frac1 6$ in the tank, so start your jumps from here.
Solution- As we are adding $\frac1 6$ + $\frac3 6$, we start at $\frac1 6$.
- Next, look at the numerator of the fraction we are adding; in $\frac3 6$ the numerator is 3 so we make 3 jumps.
- This gets us to $\frac4 6$.
- So $\frac1 6$ + $\frac3 6$ = $\frac4 6$
-
Which number lines show the correct way of adding the fractions?
HintsThe number line should be divided into equal parts based on the numerator. What is the numerator in the fractions that Axel and Tank are adding here?
The friends added $\frac1 9$ and $\frac6 9$. There are two ways to add these fractions, depending on which order they are added.
To add $\frac1 9$ and $\frac6 9$, the friends could start at $\frac1 9$ and make 6 jumps, or they could start at $\frac6 9$ and make 1 jump.
Solution- There are two correct options to add $\frac1 9$ + $\frac6 9$.
- Both correct options have the number line divided into 9 equal parts because the numerators in $\frac1 9$ + $\frac6 9$ are 9.
- To solve starting with the smaller fraction: $\frac1 9$ + $\frac6 9$, start at $\frac1 9$ and jump forward 6.
- To solve starting with the larger fraction: $\frac6 9$ + $\frac1 9$, start at $\frac6 9$ and jump forward 1.
-
Practise adding fractions.
HintsTo add the fractions on a number line, first partition the number line to the number of parts that is in the denominator.
Find the first fraction on the number line, then count forward by the numerator of the second fraction.
Can you simplify your answer by dividing the numerator and denominator by the same factor?
Solution- $\frac2 8$ + $\frac2 8$ = $\frac1 2$.
- $\frac3 6$ + $\frac1 6$ = $\frac2 3$.
- $\frac1 7$ + $\frac3 7$ = $\frac4 7$.
- $\frac3 5$ + $\frac2 5$ = 1.
-
Add the fractions on the number line.
HintsStart by locating the first fraction in the equation on the number line.
How many parts do you need to jump forward?
The numerator in the second fraction ($\frac4 7$) is 4, so jump forward 4 parts.
Solution- Start at $\frac2 7$
- As we are adding $\frac4 7$, look at the numerator of that fraction.
- The numerator of $\frac4 7$ is 4, so we make 4 jumps forward.
- This gets us to $\frac6 7$.
- So $\frac2 7$ + $\frac4 7$ = $\frac6 7$.
-
Adding and simplifying fractions.
HintsTo simplify a fraction, divide the numerator and denominator by the same factor. In this example $\frac{4}{10}$ is simplified to $\frac2 5$ by dividing both by 2.
Sometimes, it may be a fraction in the question that has already been simplified and needs expanding. For example, $\frac1 3$ can be expanded by multiplying both the numerator and denominator by 2 to get $\frac2 6$.
Solution1) This answer was correct. $\frac3 8$ + $\frac3 8$ = $\frac6 8$. Divide numerator and denominator by 2 to get $\frac3 4$.
2) This answer was correct. $\frac4 9$ + $\frac1 3$ = $\frac7 9$. First expand $\frac1 3$ by multiplying the numerator and denominator by 3 to get $\frac3 9$. $\frac4 9$ + $\frac3 9$ = $\frac7 9$.
3) This answer was incorrect. $\frac2 6$ + $\frac2 6$ = $\frac4 6$. Divide numerator and denominator by 2 to get $\frac2 3$.
4) This answer was incorrect. $\frac{4}{10}$ + $\frac{4}{10}$ = $\frac{8}{10}$. Divide numerator and denominator by 2 to get $\frac4 5$.
5) This answer was correct. $\frac{3}{12}$ + $\frac{5}{12}$ = $\frac{8}{12}$. Divide numerator and denominator by 4 to get $\frac2 3$.
6) This answer was correct. $\frac{1}{16}$ + $\frac{1}{16}$ + $\frac{2}{16}$ = $\frac{4}{16}$. Divide numerator and denominator by 4 to get $\frac1 4$.

Adding Fractions
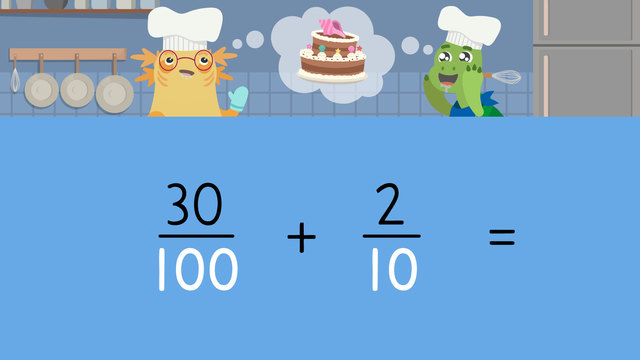
Adding Tenth and Hundredth
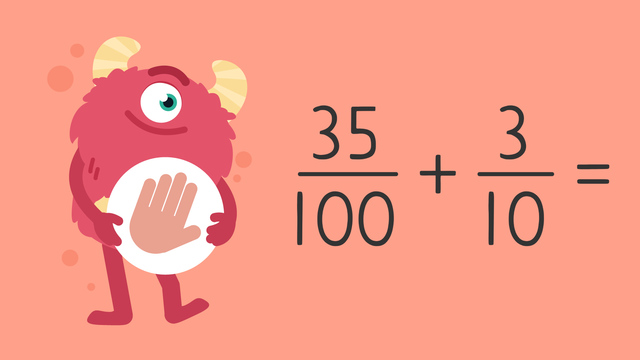
Adding Tenth and Hundredth — Let's Practise!
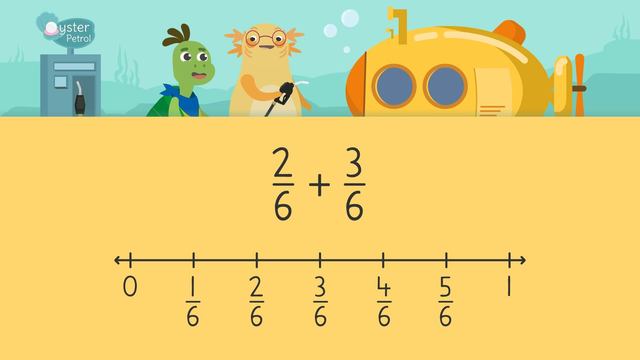
Adding Fractions on a Number Line
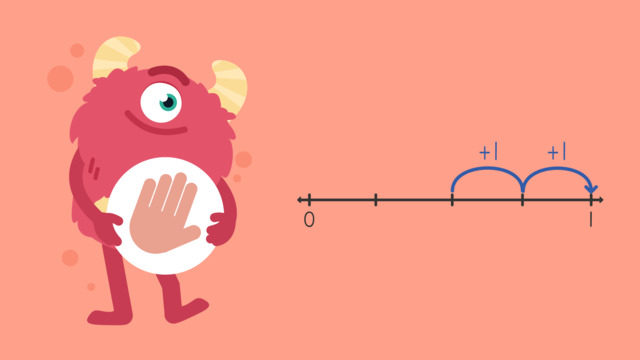
Adding Fractions on a Number Line- Let's Practise!
Subtracting Fractions on a Number Line
Subtracting Fractions on a Number Line — Let's Practise
Adding and Subtracting Mixed Numbers
Adding and Subtracting Mixed Numbers with Common Denominators—Let's Practise!

Adding Fractions with Different Denominators
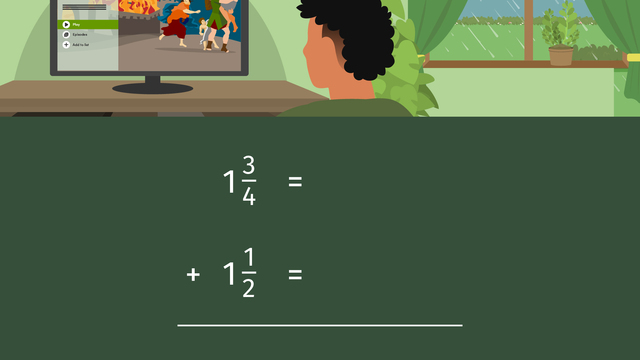
Adding Mixed Numbers with Unlike Denominators
 Do you want to learn faster and more easily?
Do you want to learn faster and more easily?








