Volume of Right Rectangular Prisms: Fractional Lengths
- Volume of a Rectangular Prism with Fractional Lengths
- Volume of a Rectangular Prism with Fractional Lengths – Explanation
- Volume of a Rectangular Prism with Fractional Lengths – Examples
- Volume of a Rectangular Prism with Fractional Lengths – Practice
- Volume of a Rectangular Prism with Fractional Lengths – Summary
- Volume of a Rectangular Prism with Fractional Lengths – Frequently Asked Questions
Learning text on the topic Volume of Right Rectangular Prisms: Fractional Lengths
Volume of a Rectangular Prism with Fractional Lengths
In daily life, understanding the volume of simple 3D shapes is crucial for tasks like organising storage or planning spaces. Furthermore, the volume of prisms is essential when structures or storage units involve fractional lengths. This knowledge helps ensure precise and efficient use of space in both personal and professional settings.
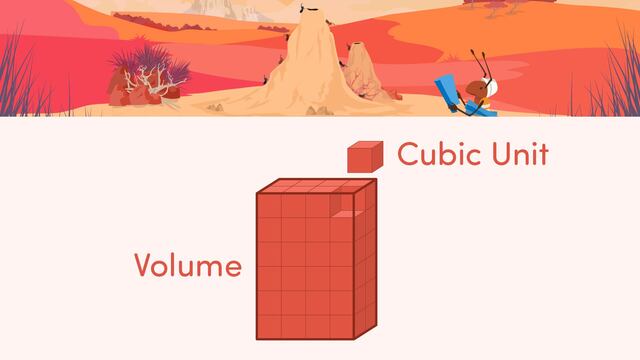
Volume is the measure of how much space an object occupies. For right rectangular prisms (a type of box shape also called a cuboid), the volume can be calculated using the formula $V = l \times w \times h$, where $l$, $w$, and $h$ are the prism's length, width, and height, respectively.
Illustration request: Show a labeled rectangular prism with length width height labeled - gotta be one in archives
Volume of a Rectangular Prism with Fractional Lengths – Explanation
The formula $V = l \times w \times h$ provides a straightforward method for calculating the volume of rectangular prisms, even when the sides are fractional lengths. Keeping dimensions in fractional form allows for precise calculations without converting into decimals, which is particularly useful in construction and engineering where exact measurements are necessary.
| Variable | Definition | Symbol |
|---|---|---|
| Length | The longest dimension of the prism | $l$ |
| Width | The dimension perpendicular to $l$ | $w$ |
| Height | The dimension from base to top | $h$ |
| Volume | The space the prism occupies | $V = l \times w \times h$ |
Volume of a Rectangular Prism with Fractional Lengths – Examples
Let’s explore how to calculate the volume with fractional dimensions using a few examples.
Example 1: Suppose a rectangular prism has dimensions $l = \frac{3}{2}$ ft, $w = \frac{5}{4}$ ft, and $h = \frac{2}{3}$ ft.
Calculate the volume:
$V = l \times w \times h = \frac{3}{2} \times \frac{5}{4} \times \frac{2}{3}$
$V = \frac{3 \times 5 \times 2}{2 \times 4 \times 3} = \frac{30}{24}$.
Simplify this to $\frac{5}{4}$ or $1 \frac{1}{4}$ft$^3$
Example 2: Suppose a rectangular prism has dimensions where the length is a mixed number: $l = 1 \frac{1}{2}$ cm, the width is a fraction: $w = \frac{4}{3}$ cm, and the height is also a fraction: $h = \frac{5}{6}$ cm.
Convert mixed number to improper fraction: Convert $1 \frac{1}{2}$ cm to an improper fraction: $ l = 1 \frac{1}{2} = \frac{3}{2} \text{ cm} $
Calculate the volume: $ V = l \times w \times h = \frac{3}{2} \times \frac{4}{3} \times \frac{5}{6} $
$ V = \frac{3 \times 4 \times 5}{2 \times 3 \times 6} = \frac{60}{36} $
Simplify this to $\frac{5}{3}$ or $1 \frac{2}{3}$ cm$^3$
Example 3: Consider another rectangular prism with a mixture of whole number, fractional, and mixed number dimensions: $l = 2$ in, $w = \frac{2}{3}$ in, and $h = 1 \frac{1}{4}$ in.
Convert mixed number to improper fraction: Convert $1 \frac{1}{4}$ in to an improper fraction: $ h = 1 \frac{1}{4} = \frac{5}{4} \text{ in} $
Calculate the volume: $ V = l \times w \times h = 2 \times \frac{2}{3} \times \frac{5}{4} $
$ V = \frac{2 \times 2 \times 5}{1 \times 3 \times 4} = \frac{20}{12} $ Simplify this to $\frac{5}{3}$ or $1 \frac{2}{3}$ in$^3$
Volume of a Rectangular Prism with Fractional Lengths – Practice
Practise finding the volume with a few more examples.
Volume of a Rectangular Prism with Fractional Lengths – Summary
Key Learnings from this Text:
- The volume of a rectangular prism can be calculated using the product of its length, width, and height.
- Using fractional measurements for volume calculations provides precise values without rounding errors common in decimal conversions.
- Understanding how to manipulate fractions is crucial for accurate volume calculations in real-world applications.
- Be sure to include the units of volume as a cubic unit.
Volume of a Rectangular Prism with Fractional Lengths – Frequently Asked Questions
Volume of Right Rectangular Prisms: Fractional Lengths exercise
-
Step by step method.
HintsStart by converting any mixed numbers to improper fractions. For example, $1 \frac{1}{2}$ becomes $\frac{3}{2}$. This will make it easier to multiply the fractions.
Remember to multiply the fractions in the order given by the formula $V = l \times w \times h$. Multiply the numerators together to get the numerator of the result, and the denominators together to get the denominator of the result.
After multiplying the fractions, simplify the resulting fraction by dividing the numerator and the denominator by their greatest common divisor. This will give you the simplest form of the volume. Final answers must also contain units too.
SolutionThe correct order is;
- Convert the mixed number to an improper fraction.
- Multiply the lengths: $\\$
- Simplify the answer fraction to give it in the form the question asks for. $\\$
- Don't forget to add the units to your final answer. They should be cubed as volume is in three dimensions.
-
Recognising dimensions.
HintsCarefully look at the diagram and find the labels for the length, width, and height of the cuboid. These labels are usually marked along the edges of the cuboid.
Take care as the orientation and angle which the prism is viewed at can change which edge may be the length, width or height.
Make sure you correctly input each dimension into the corresponding gap. The length is typically the longest side, the width is the shorter side on the same face, and the height is the vertical side.
Remember to multiply the fractions in the order given by the formula $V = l \times w \times h$. Multiply the numerators together to get the numerator of the result, and the denominators together to get the denominator of the result.
SolutionThe dimensions are:
Length: $\frac{3}{2}$ metres
Width: $\frac{5}{4}$ metres
Height: $\frac{2}{3}$ metres
Using the formula for finding the volume of a cuboid, you should use the calculation $V = \frac{3}{2} \times \frac{5}{4} \times \frac{2}{3}$. This gives a final volume of $\frac{5}{4}cm^3$.
-
Match the cuboid with the corresponding volume.
HintsBefore calculating the volume, ensure all dimensions are in fractional form. Convert any mixed numbers to improper fractions to make multiplication easier and more accurate. For example $1 \frac{1}{4} = \frac{5}{4}$.
Remember the formula for finding the volume of a rectangular prism (cuboid): $V = l \times w \times h$.
When calculating the volume, make sure to correctly multiply fractions and whole numbers. For example, to multiply $\frac{1}{2}$ by $\frac{3}{4}$, multiply the numerators ($1$ and $3$) to get $3$ and the denominators ($2$ and $4$) to get $8$, so $\frac{1}{2} \times \frac{3}{4} = \frac{3}{8}$.
SolutionThe correct pairings for this task are:
- 1) $V= 1 \frac{1}{2}ft \times 2ft \times \frac{3}{4}ft = 2\frac{1}{4}ft^3 $
- 2) $V= \frac{5}{6}m \times \frac{7}{8}m \times 1m = \frac{35}{48}m^3$
- 3) $V= 2in \times \frac{2}{3}in \times 1\frac{1}{4}in = \frac{5}{3}in^3$
- 4) $V= 1\frac{1}{2}cm \times \frac{4}{3}cm \times \frac{5}{6}cm = \frac{5}{3}cm^3$
-
Special cuboids - a cube!
HintsAll the dimensions on a cube are the same length! So the width, height and length of the cube are all $\frac{2}{5}cm^3$.
Put the dimensions into the formula to give us $Vol = \frac{2}{5} \times \frac{2}{5} \times \frac{2}{5}$.
Don't forget all volumes need units. For this question, they will be in the form $cm^3$ as the cube has dimensions in $cm$.
Another way of finding the volume of a cube is to use the formula $V = s^3$, where $s$ is the length of the side of the cube!
SolutionThe correct solution for this question is $\frac{8}{125}cm^3$.
This is worked out knowing that all the edges of a cube are the same length, so here the length, width and height will all be $\frac{2}{5}cm$. Using the formula, you will get the calculation $Vol = \frac{2}{5} \times \frac{2}{5} \times \frac{2}{5}$ which equals a total volume of $\frac{8}{125}cm^3$.
-
Calculating the volume of a cuboid.
HintsRemember the formula for the volume of a cuboid is $V = l \times w \times h$. Ensure you multiply the length, width, and height together to find the volume.
When multiplying fractions, multiply the numerators (top numbers) together and the denominators (bottom numbers) together. For example, to multiply $\frac{2}{3}$ and $\frac{4}{5}$, you calculate $\frac{2 \times 4}{3 \times 5} = \frac{8}{15}$.
After finding the product of the fractions, simplify the result if possible. This means dividing both the numerator and the denominator by their greatest common divisor. For example, $\frac{8}{12}$ can be simplified to $\frac{2}{3}$ because both $8$ and $12$ can be divided by $4$.
SolutionThe correct solution for this problem is $\frac{1}{4}cm^3$.
This is worked out using the calculation $V = \frac{3}{4}cm \times \frac{1}{2}cm \times \frac{2}{3}cm$.
The result to this calculation is $ \frac{6}{24}cm^3$, which simplifies to $\frac{1}{4}cm^3$.
-
Finding a missing dimension.
HintsRemember that the volume $V$ of a rectangular prism is calculated using the formula $V = l \times w \times h$, where $l$ is the length, $w$ is the width and $h$ is the height.
Substitute the given values into the volume formula. You'll have $\frac{3}{10} = l \times \frac{3}{5} \times \frac{6}{4}$. Rearrange the equation to solve for the missing length $l$.
To isolate $l$, divide the volume by the product of the width and height. This means you need to compute $l = \frac{3}{10} \div (\frac{3}{5} \times \frac{6}{4})$.
SolutionThe correct answer for the missing length is $\frac{1}{3}cm$.
This is calculated by substituting the values into the volume formula and then rearranging it to give us the calculation, $l = \frac{3}{10} \div (\frac{3}{5} \times \frac{6}{4})$.
By completing this you will be given a final solution of $\frac{1}{3}cm$.
 Do you want to learn faster and more easily?
Do you want to learn faster and more easily?