Volume of Rectangular Prism Using Cubic Units

Basics on the topic Volume of Rectangular Prism Using Cubic Units
Volume of Rectangular Prism Using Cubic Units
Calculating volume is a critical skill that helps us understand how much three-dimensional space an object occupies. This is especially useful for practical tasks such as determining the amount of liquid that can fill a container, the space required to store items or the capacity of a room. Grasping the volume of a rectangular prism is a fundamental concept in geometry that applies to both simple and complex real-world problems.
Understanding Volume of Rectangular Prism
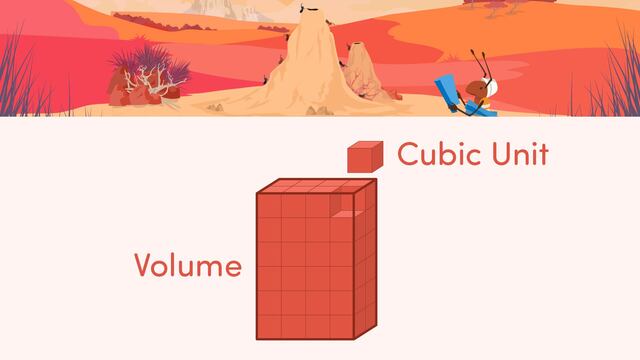
Rectangular prisms are a type of polyhedron with six faces, all of which are rectangles. Commonly referred to as a box or cuboid, these shapes are characterised by having three dimensions: length, width and height.
The volume of a rectangular prism is the measure of space within the prism and is calculated by multiplying the prism's length, width and height.
Volume of Rectangular Prism – Example
Calculating the volume of a rectangular prism is straightforward once you know the dimensions of the object. Let's go through an example for clarity.
Find the volume of a rectangular prism with a length of 8 cm, width of 3 cm and height of 5 cm.
- Identify the dimensions: Length = 8 cm, Width = 3 cm, Height = 5 cm.
- Apply the volume formula: Volume = Length x Width x Height.
- Calculate the volume: Volume = 8 cm x 3 cm x 5 cm.
- Result: The volume of the rectangular prism is 120 cubic centimetres.
Real-World Applications of Volume
Understanding how to calculate the volume of a rectangular prism has numerous practical applications:
- Packaging industries use volume to determine box sizes for shipping goods.
- In construction, volume calculations help in material estimation for concrete slabs, foundations and walls.
- Interior designers calculate the volume of rooms to optimise heating and cooling systems.
Volume of a Rectangular Prism – Practice
Common Mistakes to Avoid
- Inconsistent units: Always ensure that all measurements are in the same unit before calculating volume.
- Rounding off too early: Round off the final answer to avoid accumulation of errors.
- Neglecting to double-check: Always verify measurements and calculations to avoid mistakes.
Volume of Rectangular Prism Using Cubic Units – Summary
Key Learnings from this Text:
Volume calculation is important for understanding the space occupied by three-dimensional objects.
The formula for the volume of a rectangular prism is Volume = Length x Width x Height.
- Real-world applications of volume are extensive and span many industries.
- Consistency and accuracy in measurements are crucial for correct volume determination.
Expand your skills in geometry and measurement with additional resources, interactive practice problems and videos available on our educational platform. For more, have a look at the formula for the volume of a cuboid.
Volume of Rectangular Prism – Frequently Asked Questions
Transcript Volume of Rectangular Prism Using Cubic Units
In a bustling insect world where the ant population was booming, an industrious builder took on a remarkable challenge: constructing an apartment complex for the growing ant community. Armed with blueprints, the builder was tasked with determining the space's volume and ensuring that each apartment would accommodate the growing number of ants. He will need to find the volume of rectangular prisms using unit cubes. A rectangular prism is a three-dimensional shape with six faces, all rectangles. We also call this shape a cuboid. The volume of a rectangular prism refers to the measurement of the space inside the shape, specifically the capacity, or how much it can hold. A cubic unit measures a figure's three sides: its length, width, and height. Each side has a measurement of one cubic unit. We can determine the volume of a rectangular prism by counting the number of cubes that fill the space. Let's find the volume of this shape. First, let's see how many unit cubes are along the length of the prism. There are three. Next, count how many units are along the width. There are five. Now, count the number of cubes that make up the height. Four. We can split this prism into the layers and see that each layer has the same amount. To find the volume we can count the number of cubes in one layer and multiply that by the number of layers. Therefore, we are multiplying the length times the width times the height. We can call this layer the base of the prism. How many cubic units are in the base? Fifteen. Multiply fifteen by four. Sixty. This prism has the volume of sixty cubic units. Here are some examples to try on your own, pause the video whenever you need extended time. What is the volume of this rectangular prism? Here, the length is two cubic units. The width is four. And the height is two. If we multiply the length times the width times the height we get the volume of sixteen cubic units. What is the volume of this rectangular prism? The length is two, the width is five, and the height is three. The volume is thirty cubic units. Match the rectangular prisms with their volume. Number one is ,C. Two is A. Three is D, and four is B. At the building site, the diligent ants use cubic units to measure the volume of space. This helps them determine how many ants can fill each area within the apartment complex, ensuring a comfortable living environment for their community.
Volume of Rectangular Prism Using Cubic Units exercise
-
What is the volume of this rectangular prism?
HintsTo find the volume, we must multiply the length by the width by the height.
Count the cubes along each side to find these measurements.
Here we can see the length is 4 units, the width is 5 units and the height is 6 units.
Multiply 4 x 5 x 6 to find the volume.
SolutionThe correct answer is 120 cubic units.
- The length of the rectangular prism is 4 units.
- The width of the rectangular prism is 5 units.
- The height of the rectangular prism is 6 units.
4 x 5 x 6 = 120
-
Can you find the volume of each rectangular prism?
HintsRemember, to find the volume we use this formula.
- $V$ = volume
- $l$ = length
- $w$ = width
- $h$ = height
Count the blocks on each rectangular prism to find the length, width and height.
For this rectangular prism, the length is 5 units, the height is 1 unit and the width is 3 units. Multiply these together to find the volume.
SolutionVolume of A = 15 cubic units
- length = 5 units
- height = 1 unit
- width = 3 units
- 5 x 1 x 3 = 15
- length = 7 units
- height = 3 unit
- width = 2 units
- 7 x 3 x 2 = 42
- length = 6 units
- height = 3 unit
- width = 3 units
- 6 x 3 x 3 = 54
- length = 8 units
- height = 1 unit
- width = 3 units
- 8 x 1 x 3 = 24
-
What is the volume of these rectangular prisms?
HintsUse this formula to find each volume where $l$ = length, $w$ = width and $h$ = height.
You could draw out a rectangular prism if it helps you.
For example, we could use this one to find the volume for the prism with the length of 5 units, width of 3 units and height of 2 units.
SolutionVolume of 30 cubic units
length = 5 units; width = 3 units; height = 2 units
- 5 x 3 x 2 = 30
- 10 x 3 x 1 = 30
length = 6 units; width = 4 units; height = 2 units
- 6 x 4 x 2 = 48
- 4 x 3 x 4 = 48
length = 7 units; width = 3 units; height = 4 units
- 7 x 3 x 4 = 84
- 7 x 6 x 2 = 84
-
Can you order these prisms?
HintsUse this formula to find each volume where $l$ = length, $w$ = width and $h$ = height.
You could draw out a rectangular prism if it helps you.
For example, we could use this one to find the volume for the prism with the length of 7 units, width of 4 units and height of 2 units.
Work out all of the volumes and then order the measurements from smallest to biggest.
Solution1.) $l$ : 6 units, $w$ : 1 unit, $h$ : 5 units
- 6 x 1 x 5 = 30
- 6 x 4 x 2 = 48
- 7 x 4 x 2 = 56
- 5 x 4 x 3 = 60
- 8 x 3 x 6 = 144
-
What is the volume of the rectangular prism?
HintsMultiply the length by the width by the height to find the volume.
The length is 4 units.
The width is 3 units.
The height is 2 units.
Multiply these together to find the volume.
SolutionThe correct answer is 24 cubic units.
The length is 4 units.
The width is 3 units.
The height is 2 units.
4 x 3 x 2 = 24
-
How many cereal boxes will fit into the box?
HintsFirst, work out the volume of one cereal box.
20 x 5 x 30 = ?
Next, work out the volume of the larger box.
60 x 20 x 40 = ?
To make this calculation easier, solve 6 x 2 x 4 and then add three zeros back on.
Now divide the volume of the larger box by the volume of one cereal box.
SolutionThe correct answer is 16 cereal boxes.
The volume of one cereal box is 20 x 5 x 30 = 3,000 centimetres cubed
The volume of the larger box is 60 x 20 x 40 = 48,000 centimetres cubed
48,000 $\div$ 3,000 = 16
 Do you want to learn faster and more easily?
Do you want to learn faster and more easily?













