Volume of Simple 3D Shapes
Learning text on the topic Volume of Simple 3D Shapes
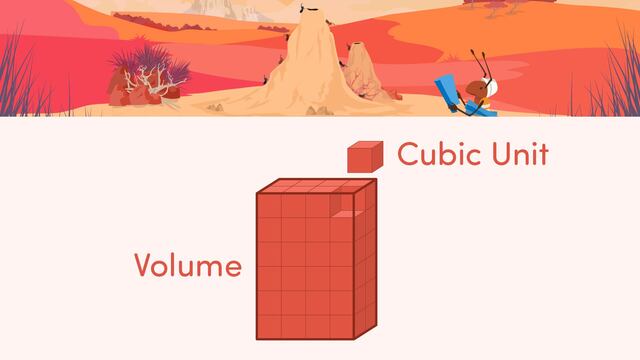
Volume of a 3D Shape
Volume is a fundamental concept in geometry and everyday life, allowing us to quantify the three-dimensional space occupied by an object. From filling a swimming pool to baking a cake, understanding volume is essential for a myriad of activities.
Understanding Volume – Definition
Volume is the measure of the amount of space an object occupies in three dimensions. It’s commonly measured in cubic units, such as cubic centimetres (cm$^3$) or cubic feet (ft$^3$).
In terms of mathematics, volume is a property of three-dimensional shapes, and calculating it often requires knowledge of certain formulas that apply to these shapes. There is a large range of 3D shapes you may need to find the volume of, but we are going to focus on prisms for the time being. A prism is defined as a solid object with two identical ends and flat sides. If you cut through a prism you will always see the same cross-section shape. Below is an example of a rectangular prism, also known as a cuboid.
Volume Formulas for 3D Shapes – Examples
There are all different types of 3D shapes and each of them uses a different formula to calculate the volume. Here is a table showing some common 3D shapes, what they look like and the formula used to find their volume.
| Shape | Description | Volume Formula | Illustration Request |
|---|---|---|---|
| Rectangular Prism | A box-shaped figure | $V = l \times w \times h$ |  |
| Cube | A six-sided figure with equal sides | $V = s^{3}$ | 
|
| Triangular Prism | A prism with triangular bases | $V = \frac{1}{2} \times b \times h \times l$ |  |
| Cylinder | A circular solid figure | $V = \pi \times r^{2} \times h$ |
 |
Volume of a Rectangular Prism
Example: Find the volume of a rectangular prism with a length of $10$ cm, a width of $4$ cm, and a height of $5$ cm.
Solution:
Identify the dimensions: Length = $10$ cm, Width = $4$ cm, Height = $5$ cm.
Apply the volume formula for a rectangular prism: Volume = Length x Width x Height.
Calculate: Multiply the dimensions: $10$ cm x $4$ cm x $5$ cm = $200$ cm³.
Result: The volume of the rectangular prism is $200$ cm³.
Volume of a Cube
Example: Find the volume of a cube with sides of length $3$ cm.
Solution:
Identify the length of the side: The side length is $3$ cm.
Apply the volume formula for a cube: Volume = Side$^{3}$.
Calculate: Cube the side length: $3$ cm x $3$ cm x $3$ cm = $27$ cm$^{3}$.
Result: The volume of the cube is $27$ cm$^{3}$.
Volume of a Triangular Prism
Example: Find the volume of a triangular prism with a base of 6 cm, a height of the triangle of $4$ cm, and a length of $10$ cm.
Solution: 1. Identify the dimensions: Base = $6$ cm, Height of the triangle = $4$ cm, Length = $10$ cm.
Apply the volume formula for a triangular prism: $Volume = \frac{1}{2} \times \text{Base} \times \text{Height of the triangle} \times \text{Length}$.
Calculate: Multiply the dimensions: $\frac{1}{2} \times 6 \text{ cm} \times 4 \text{ cm} \times 10 \text{ cm} = 120 \text{ cm}^{3}$.
Result: The volume of the triangular prism is $120$ cm³.
Volume of a Cylinder
Example: Find the volume of a cylinder with a radius of $5$ cm and a height of $7$ cm.
Solution:
Identify the dimensions: Radius = $5$ cm, Height = $7$ cm.
Apply the volume formula for a cylinder: Volume = $\pi \times \text{Radius}^2 \times \text{Height}$.
Calculate: Multiply the dimensions: $\pi \times (5 \text{cm})^{2} \times 7 \text{cm} \approx 549.78 \text{cm}^{3}$.
Result: The volume of the cylinder is approximately $549.78$ cm³.
Volume of Simple 3D Shapes – Practice
Volume of Simple 3D Shapes – Summary
Key Learnings from this Text:
- Volume measures the space occupied by a 3D object and is expressed in cubic units.
- For simple 3D shapes, specific formulas allow us to calculate their volume.
- These principles have practical applications in various fields from engineering to daily tasks.
- Understanding and calculating volume is crucial for efficient space management.
For more, check out the formula for the volume of a cuboid as well as our video on the volume of a cylinder.
Volume Formulas for Simple 3D Shapes – Frequently Asked Questions
Volume of Simple 3D Shapes exercise
-
Match the volume formula to the correct 3D shape.
HintsLook at the images of the 3D shapes. They have dimensions marked on them using letters to represent things like length, width, height and the radius. You can use these dimension letters to help you find the correct corresponding volume formulas.
For the rectangular prism, or cuboid, think about the shape of a typical box. You need to multiply its length, width, and height to find its volume.
For the cube, remember that all its sides are equal. The formula involves cubing the length of one of its sides or multiplying it's side by itself three times.
For the cylinder, visualise it as a can. Its volume formula includes π (pi), the radius of the base (which is a circle) and its height.
For the triangular prism, imagine a tent shape. The volume formula involves finding the area of the triangular face first (using $\frac{1}{2} \times \text{base} \times \text{height}$) and then multiplying by the length of the prism.
SolutionThe correct pairs are:
- Cuboid - $V = l \times w \times h$
- Cube - $V = s \times s \times s$
- Triangular Prism - $V = \frac{1}{2} (b \times h) \times l$
- Cylinder - $V = π r^2$
-
Which 3D shape has the largest volume?
HintsFor the cube, remember that all sides are the same length. To find its volume, you need to cube the side length, which means multiplying the side length by itself three times. The formula is $ \text{Volume} = \text{Side}^3 $.
For the cuboid, you need to identify the three different dimensions: length, width, and height. To find its volume, multiply these three dimensions together. The formula is $ \text{Volume} = \text{Length} \times \text{Width} \times \text{Height} $.
After calculating the volumes, compare the two results. The one with the larger number is the shape with the bigger volume. Make sure to double-check your multiplication to ensure accuracy in comparing the two volumes.
SolutionVolume of the Cube:
The formula for the volume of a cube is: Volume = Side³.
Identify the side length of the cube: Side = $5cm$.
Calculate the volume of the cube: Volume = $125cm^3$.
Volume of the Cuboid:
The formula for the volume of a cuboid is: Volume = Length × Width × Height.
Identify the dimensions of the cuboid: Length = $6cm$, Width = $4cm$, Height = $3cm$.
Calculate the volume of the cuboid: Volume = $72cm^3$.
Comparison:
The volume of the cube is $125cm^3$ and the volume of the cuboid is $72cm^3$.
Therefore, the volume of the cube is bigger
-
Position the words and numbers into the gaps.
HintsTo find the volume of a triangular prism, you need to first calculate the area of the triangular base. Use the formula for the area of a triangle, which is $ \frac{1}{2} \times b \times h$.
Once you have the area of the triangle, multiply it by the length (or depth) of the prism to get the volume. This is because the volume of a prism is the area of the base shape times the length of the prism.
Be sure to match the correct dimensions to their labels: the base and height are for the triangle, and the length is the distance between the triangular bases. Remember to use $\frac{1}{2}$ in your calculation for the area of the triangle.
Solution- Identify the dimensions: Base = 6 cm, Height of the triangle = 4 cm, Length = 10 cm.
- Apply the volume formula for a triangular prism: Volume = $\frac{1}{2}$ × b × h × l.
- Calculate: Multiply the dimensions: $\frac{1}{2} \times$ 6 × 4 × 10 = 120.
- Result: The volume of the triangular prism is 120 cm³. It's important to remember the units too.
-
Calculate the volumes of the 3D shapes.
HintsFor the rectangular prism, think about the shape of a typical box. You need to multiply its length, width and height to find its volume.
For the cube, remember that all its sides are equal. The formula involves cubing the length of one of its sides.
For the cylinder, visualise it as a can. Its volume formula includes π (pi), the radius of the base (which is a circle) and its height.
For the triangular prism, imagine a tent shape. The volume formula involves finding the area of the triangular base first (using $\frac{1}{2} \times \text{base} \times \text{height}$ of the triangle) and then multiplying by the length of the prism.
SolutionThe correct volumes for each shape are:
- Rectangular Prism: $V =180 cm³$
- Cube: $V = 64 cm³$
- Cylinder: $V = 141.3 cm³$
- Triangular Prism: $V = 120 cm³$
-
What is the volume of a cube with a side length of 4 cm?
HintsA cube has all its sides of equal length. To find the volume, you need to use the formula for the volume of a cube: $V = s \times s \times s$, where $s$ is the length of one side.
Since all sides of a cube are the same length, you need to multiply the side length by itself three times. For example, if the side length is 4 cm, calculate $ 4 \times 4 \times 4 $.
SolutionThe correct volume for a cube with sides $4 \:cm$ is $64\:cm^3$.
This is calculated using the formula $V= s \times s \times s$ where $s$ is the side length. Therefore, the volume is calculated by $V = 4 \times 4 \times 4$ and gives us the solution of $64 \:cm^3$.
-
Sort the 3D shapes.
HintsFor the cube, remember that all sides are equal. To find its volume, use the formula, $ \text{Volume} = s^3 $. In this case, you need to calculate $ 3 \, \text{cm} \times 3 \, \text{cm} \times 3 \, \text{cm} $.
For the cuboid, identify the three different dimensions: length, width, and height. The volume formula is $ \text{Volume} = l \times w \times h$. Plug in the values $ 4 \, \text{cm} \times 3 \, \text{cm} \times 2 \, \text{cm} $ to find the volume.
For the triangular prism, you need to find the area of the triangular face first using the formula $ \frac{1}{2} \times b \times h $, then multiply this area by the length of the prism.
For the cylinder, use the formula $ \text{Volume} = \pi \times r^2 \times h$. Remember that $\pi$ is approximately 3.14.
When comparing volumes, the bigger number represents the larger volumes. For example, $45cm^3$ is bigger in volume than $28cm^3$.
SolutionCalculate the volumes: $\\$ 1. Volume of the Cube = Side³ = $3 cm³$ = $27 cm³$ $\\$ 2. Volume of the Cuboid = Length × Width × Height = $4 cm$ × $3 cm$ × $2 cm$ = $24 cm³$ $\\$ 3. Volume of the Triangular Prism = ½ × Base × Height of the triangle × Length = ½ × $4 cm$ × $3 cm$ × $5 cm$ = $30 cm³$ $\\$ 4. Volume of the Cylinder = π × Radius² × Height = π × $2 cm$² × $4 cm$ ≈ $50.24 cm³$ (use π ≈ 3.14) $\\$
Order the shapes from smallest to largest volume: $\\$ 1. Cuboid: $24 cm³$ $\\$ 2. Cube: $27 cm³$ $\\$ 3. Triangular Prism: $30 cm³$ $\\$ 4. Cylinder: $50.24 cm³$ to 2 decimal places $\\$
 Do you want to learn faster and more easily?
Do you want to learn faster and more easily?