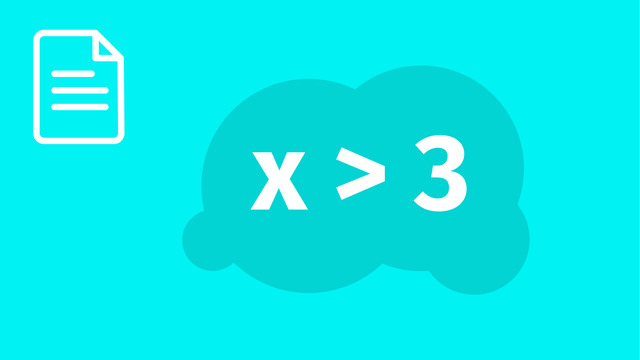
Writing Equivalent Algebraic Expressions with Addition and Subtraction
- Writing Equivalent Algebraic Expressions with Addition and Subtraction – Definition
- Understanding Equivalent Algebraic Expressions – Explanation
- Like Terms
Learning text on the topic Writing Equivalent Algebraic Expressions with Addition and Subtraction
Writing Equivalent Algebraic Expressions with Addition and Subtraction – Definition
When we talk about algebra, we often find different expressions that can represent the same value or idea. This concept is crucial not just in mathematics but in solving problems in everyday life where flexibility and adaptation to various representations can lead to easier solutions. An equivalent algebraic expression is an expression that, although it may look different, has the same value as another expression when the variable(s) are substituted with the same number(s).
Equivalent algebraic expressions are different algebraic expressions that represent the same value.
Understanding Equivalent Algebraic Expressions – Explanation
To master the art of writing equivalent expressions, a fundamental skill in algebra, it's essential to understand not just the process of adding and subtracting expressions effectively, but also to deeply comprehend the components that make up algebraic terms and expressions. This comprehension includes recognising and distinguishing between variables, constants, and coefficients, in addition to identifying like terms. Engaging with writing numerical expressions can significantly enhance your ability to manipulate and understand these algebraic concepts.
| Word | Definition | Example |
|---|---|---|
| Variable | A symbol representing unknown values that can change. | In $2x + 3$, $x$ is the variable. |
| Constant | A fixed value that does not change. | In $2x + 3$, $3$ is the constant. |
| Coefficient | A numerical factor that multiplies a variable in an algebraic expression. | In $2x + 3$, $2$ is the coefficient of $x$. |
| Term | A single mathematical expression consisting of a constant, variable, or both, possibly multiplied together. | $2x$ and $3$ are both terms in the expression $2x + 3$. |
Like Terms
Like terms are terms in an algebraic expression that have the same variable raised to the same power. The process of simplifying an expression by adding or subtracting like terms is known as combining like terms. This is a foundational skill for working with algebraic expressions.
Adding and Subtracting Algebraic Expressions
Adding or subtracting algebraic expressions involves combining like terms by adding or subtracting their coefficients. This method is straightforward yet powerful, allowing us to simplify complex expressions into more manageable forms.
Simplify $4x + x$
- Identify like terms: In the expression $4x + x$, both terms are like terms because they contain the same variable, $x$.
- Add the coefficients of like terms: The coefficient of $x$ in the term $x$ is $1$ (since $x$ is the same as $1x$). Therefore, when adding $4x$ and $x$, you are essentially adding $4x + 1x$.
- Perform the addition: Add the coefficients $4$ and $1$ to get $5$.
- Write the simplified expression: After adding the coefficients, the simplified expression is $5x$.
Simplify $7n + 3m - 5n + m$
- Identify like terms: In the expression $7n + 3m - 5n + m$, $7n$ and $-5n$ are like terms because they both contain the variable $n$. Similarly, $3m$ and $m$ are like terms because they both contain the variable $m$.
- Add the coefficients of like terms: The coefficients of $n$ terms are $7$ and $-5$, and for $m$ terms are $3$ and $1$ (since $m$ is the same as $1m$).
- Perform the addition: Add the coefficients of $n$ terms $7$ and $-5$ to get $2$, and add the coefficients of $m$ terms $3$ and $1$ to get $4$.
- Write the simplified expression: After adding the coefficients, the simplified expression is $2n + 4m$.
Simplify $-6w + w - k + 2k$
- Identify like terms: In the expression $-6w + w - k + 2k$, $-6w$ and $w$ are like terms because they both contain the variable $w$. Similarly, $-k$ and $2k$ are like terms because they both contain the variable $k$.
- Add the coefficients of like terms: The coefficients for $w$ terms are $-6$ and $1$ (since $w$ is the same as $1w$), and for $k$ terms are $-1$ (since $k$ is the same as $-1k$) and $2$.
- Perform the addition: Add the coefficients of $w$ terms $-6$ and $1$ to get $-5$, and add the coefficients of $k$ terms $-1$ and $2$ to get $1$.
- Write the simplified expression: After adding the coefficients, the simplified expression is $-5w + k$.
Practice combining like terms on your own now.
Writing Equivalent Algebraic Expressions – Application
Example 1: Imagine participating in a month-long fitness challenge. In the first week, you plan to complete $x$ minutes of cardio and double that amount in strength training. In the second week, you aim to increase your cardio by $30$ minutes while keeping strength training constant. How can you express the total minutes spent on each activity over the two weeks?
Expression:
- First week cardio: $x$ minutes.
- First week strength training: $2x$ minutes.
- Second week cardio: $x + 30$ minutes.
- Total minutes on cardio over two weeks: $x + (x + 30)$.
- Total minutes of strength training over two weeks: $2x$ (no change in the second week).
Combining Like Terms:
- Total cardio minutes: $2x + 30$.
- Total strength training minutes: $2x$.
Example 2: You're working on an art project that requires blue and yellow paint. On the first day, you use $b$ ounces of blue paint and $y$ ounces of yellow paint. The next day, inspired, you decide to double your usage of blue paint but use half the amount of yellow paint. How can you express the total paint used?
Expression:
- First day blue paint: $b$ ounces.
- Second day blue paint: $2b$ ounces.
- Total blue paint used: $b + 2b$.
- First day yellow paint: $y$ ounces.
- Second day yellow paint: $\frac{y}{2}$ ounces.
- Total yellow paint used: $y + \frac{y}{2}$.
Combining Like Terms:
- Total blue paint: $3b$ ounces.
- Total yellow paint: $\frac{3y}{2}$ ounces.
Example 3: Your book club decides to read $n$ pages of a novel each week. However, during the second week, the club agrees to read an additional $10$ pages to finish earlier. How can you express the total pages read after two weeks?
Expression:
- Week 1 pages: $n$.
- Week 2 pages (with additional): $n + 10$.
Combining Like Terms:
- Total pages read after two weeks: $2n + 10$.
Try some on your own!
Writing Equivalent Algebraic Expressions with Addition and Subtraction – Summary
Key Learnings from this Text:
- Equivalent algebraic expressions show the same value, useful in algebra and daily problem-solving.
- Like terms share the same variable and power, vital for the simplification process.
- A coefficient is a number multiplying a variable; a constant lacks a variable.
- Simplifying expressions by adding/subtracting like terms aids in managing complex algebra problems.
Writing Equivalent Algebraic Expressions with Addition and Subtraction – Frequently Asked Questions
Writing Equivalent Algebraic Expressions with Addition and Subtraction exercise
-
Write equivalent algebraic expressions in a real world context.
HintsWe can represent $3$ cars, $c$, as $3c$.
We can represent $13$ flowers, $f$, and $1$ tree, $t$, as $13f + 1t$.
Solution- Dan has $3$ cakes, $c$, and $4$ donuts, $d$. We can write this using the variable. $3$ cakes, $c = 3c$ and $4$ donuts, $d = 4d$. The expression is $3c + 4d$.
- Sam sells $2$ bikes, $b$, and $7$ scooters, $s$. We can write $2b + 7s$.
- Ali has $6$ aunties, $a$, and $5$ uncles, $u$. We can write $6a + 5u$.
-
Write an equivalent algebraic expression with addition.
HintsAddition is commutative. We can add in any order and the answer is the same. For example, $2 + 3 = 3 + 2$.
If the terms have the same variable, then we can add the coefficients. For example, $2t + t = (t + t) + t = 3t$.
We can add terms together if they are the same variable and the same power. For example, $2w + 3w = 5w$, but $2w + 3g$ is still $2w + 3g$ and cannot be simplified further.
When there are two different variables in one expression, we would simplify it like the example below.
$2w + 3r + 4w + 5r$
$= 2w + 4w + 3r + 5r$
$= 6w + 8r$
Solution- $2p + 3e + 4p + 2e = 6p + 5e$ (See image)
- $3p + 4e + 4p + 2e = 7p + 6e$
- $5p + 3e + 2p + 2e = 7p + 5e$
- $3p + 3e + 3p + 4e = 6p + 7e$
-
Simplify the expression.
HintsAs addition is commutative, it is easier to move the like terms around in variable pairs. For example, $(3a + 3a) + (2b + 6b) + (4c + c)$.
We do not write the coefficient $1$ in front of the variable, for example, $1c = c$. Therefore, $7c + c = 8c$.
Solution$6a + 8b + 5c$
- Rearrange the terms into variable pairs.
- Add the coefficients.
- $3a + 3a + 2b + 6b + 4c + c$
- $3a + 3a = 6a$
- $2b + 6b = 8b$
- $4c + c = 5c$
-
Write an equivalent algebraic expression with subtraction.
HintsTo simplify using subtraction, we rearrange the expression into pairs using addition, as it is commutative.
Add the $a$'s together and the $b$'s together.
For example:
- $6a + (-4a) = 6a - 4a = 2a$
SolutionAnswer is $2a + 3b$
-
Write an equivalent algebraic expression.
HintsIf we do not know Luke's age, we can use a variable. A variable is a quantity that can be changed, so we write $n$ for Luke's age. Jamie is $4$ years older than Luke, so we add the $4$ to form an expression.
If Luke was $n$ years old and Jamie was $10$ years older, we could write Jamie's age as $n + 10$.
Solution$n + 4$
- Luke is $n$ years
- Jamie is $4$ years older than Luke, (that is $+4$)
- Jamie is $n + 4$.
-
Form and simplify the expression.
HintsIf I have $5$ melons and $2$ are bad, I could write an expression for the good ones using the variable, $m$.
$5m - 2m$.
$9$ apples and $7$ pears. If $2$ of the apples are bad and $3$ of the pears are bad. We form an expression using the variable $a$ for apples and $p$ for pears.
$9a + 7p - 2a - 3p$
When we have the expression we:
- rearrange into variable pairs
- use addition to simplify.
Solution- $9a + 7p - 2a - 3p$
- $9a + (-2a) + 7p + (-3p)$
- $9a - 2a + 7p - 3p$
- $7a + 4p$
 Do you want to learn faster and more easily?
Do you want to learn faster and more easily?