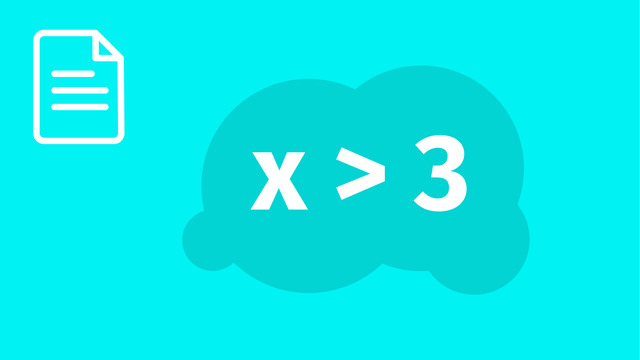
Solving Real-World Problems Using Equations
Do you want to learn faster and more easily?
Learning text on the topic Solving Real-World Problems Using Equations
Solving Real World Problems Using Equations
Equations are mathematical tools that we use to find unknown values, called variables, by setting them equal to known values. They are extremely useful in real-life situations, such as budgeting, planning, and problem-solving. Solving one-step equations is a commonly used maths skill used in the world.
In mathematics, solving equations involves finding the values of variables that make the equation true. One-step equations, where all terms are positive, provide a straightforward method to solve real-world problems without complexity.
Solving Real World Problems Using Equations – Definitions
Understanding the components of an equation is crucial, especially with writing linear equations. Here's a breakdown of the different parts of an equation:
| Term | Definition |
|---|---|
| Variable | A symbol used to represent an unknown number. |
| Constant | A number that doesn’t change within the equation. |
| Coefficient | A number used to multiply a variable. |
Solving Real-World Problems Using Equations – Step-by-Step Process
To write an equation from a word problem, identify what you need to find, what you know, and what the problem is asking. The goal is to set up an equation where the variable represents the unknown quantity you're solving for.
Example 1
Lucy has $£6$ and receives some more money as a gift. If she now has $£18$, how much money did she receive?
* Identify a variable: Let $ x $ represent the amount of money Lucy received.
* Determine the operation and write the equation: $ 6 + x = 18 $
* Solve the equation by performing the inverse operation: $ x = 18 - 6 $
* Share the solution: Lucy received $£12$.
Example 2
Mark had $£9$ but spent some on a snack. If he now has $£4$ left, how much did he spend?
* Identify a variable: Let $ x $ be the amount Mark spent.
* Determine the operation and write the equation: $ 9 - x = 4 $
* Solve the equation by performing the inverse operation: $ x = 9 - 4 $
* Share the solution: Mark spent $£5$.
Example 3
A box of crayons costs $£7$. If Tom spent $£42$ on crayons, how many boxes did he buy?
* Identify a variable: Let $ x $ be the number of boxes Tom bought.
* Determine the operation and write the equation: $ 7x = 42 $
* Solve the equation by performing the inverse operation: $ x = \frac{42}{7} $
* Share the solution: Tom bought $6$ boxes.
Example 4
Sarah divides her collection of $£15$ stickers equally among her 5 friends. How many stickers does each friend get?
* Identify a variable: Let $ x $ be the number of stickers each friend gets.
* Determine the operation and write the equation: $ 15 = 5x $
* Solve the equation by performing the inverse operation: $ x = \frac{15}{5} $
* Share the solution: Each friend gets $3$ stickers.
Try some exercises on your own following this process.
Solving Real-World Problems Using Equations – Summary
Key Learnings from this Text:
- Equations allow for systematic problem-solving by identifying unknowns in real-life scenarios.
- One-step equations are accessible for beginners, providing a clear path from problem to solution without the complexity of negatives.
- Understanding and using the components of an equation (variables, constants, coefficients) is essential for setting up correct equations.
Solving Real-World Problems Using Equations – Frequently Asked Questions
