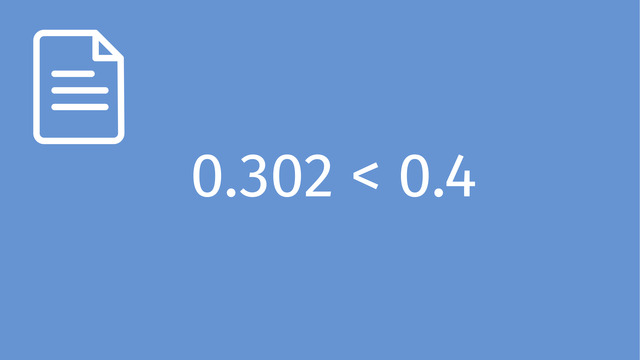Comparing Decimals using Place Value
- Comparing Decimals using Place Value – Introduction
- Understanding Decimal Place Value – Definition
- Comparing Place Value Decimals – Example
- Comparing Place Value Decimals – Guided Practice
- Comparing Place Value Decimals – Practice
- Comparing Decimals using Place Value – Summary
- Comparing Decimals using Place Value – Frequently Asked Questions
Learning text on the topic Comparing Decimals using Place Value
Comparing Decimals using Place Value – Introduction
When we deal with numbers, each digit's position can dramatically change its value. This is especially true with decimal numbers, where the concept of place value becomes quite important. Understanding and comparing place value decimals is crucial, as it sets the foundation for mathematical precision and accuracy in real-world applications.
Understanding Decimal Place Value – Definition
The place value of a decimal number determines the value of the digit based on its position relative to the decimal point. To the left of the decimal point are whole numbers, and to the right are fractional parts.
The further left a digit is, the greater its value. Moving rightwards from the decimal point, each place represents a fraction of a whole, decreasing in value by a factor of ten. Using a place value chart can help you to visualise the positioning and hence value of each digit in a number.
Comparing Place Value Decimals – Example
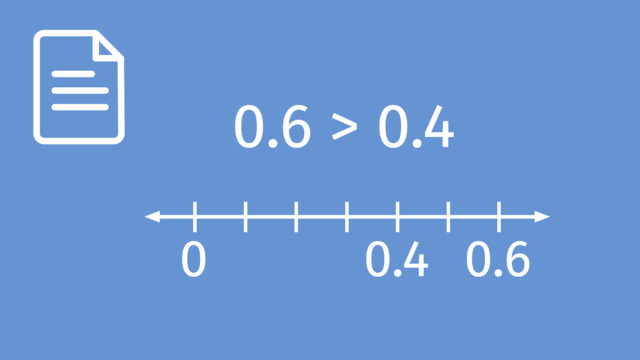
Learning to compare decimals is like sizing up different objects to see which is heavier, longer, or taller. The same logic applies to numbers, where we compare place values to determine which decimal is greater, lesser, or if they are equal.
Here's how to compare two decimals:
- Step 1: Look at the whole number part before the decimal point. The larger whole number indicates a larger value.
- Step 2: Compare the digits after the decimal point starting with the Tenths place. If they are the same, move to the next place value.
- Step 3: Continue comparing digits until you find a place where the digits differ. The decimal with the larger digit in the smallest place value where they differ is the greater number.
2.403 < 2.41
Comparing Place Value Decimals – Guided Practice
Comparing Place Value Decimals – Practice
Practice comparing decimals independently!
Comparing Decimals using Place Value – Summary
Key Learnings from this Text:
- Decimal place value is essential for understanding the worth of numbers in different positions.
- To compare decimals, start with the whole number part, then move rightward, comparing digits until they differ.
- A place value chart is a helpful tool for visualising and understanding decimals.
- Real-world applications of comparing decimals include financial literacy, measurements in science, and more.
Comparing Decimals using Place Value – Frequently Asked Questions
Comparing Decimals using Place Value exercise
-
What does the digit '4' represent in the decimal number 3.48?
HintsThe digit is directly to the right of the decimal point.
Each position to the right of the decimal point represents a fraction of ten, with the first position being tenths, and the second hundredths.
In the number 7.12, the 1 represents one tenth.
Use this to help you find the value of 4 in 3.48.
SolutionThe digit 4 in the decimal number 3.48 represents 4 tenths, or 0.4.
-
Why is 1.5 greater than 1.49?
HintsWhen comparing numbers, we start by looking at the greatest place value which is on the left.
Look at the first digit after the decimal point in each number.
How do these digits compare?
Compare the two numbers.
Solution1.5 is greater than 1.49 because when comparing decimals, the digits are compared place by place from left to right.
In the tenths place, 1.5 has a 5 whereas 1.49 has an 4.
Since 5 is greater than 4, 1.5 is greater than 1.49.
-
If you were to compare 0.701 and 0.72, which decimal would be greater and why?
HintsAdd an extra zero to the end of 0.72 to compare it directly with 0.701.
Does this change the way you compare the two decimals?
Remember to compare from left to right.
What are the values in the hundredths place for each decimal?
The final step would be to compare the thousandths place but is that necessary with these numbers?
Solution0.72 is greater than 0.701 because in the hundredths place, 0.72 has a 2 while 0.701 has a 0, and since the hundredths place is the first place where they differ, the number with the higher digit in this place —0.72— is the greater decimal.
1: Align the decimals to compare them directly. Write them as 0.720 and 0.701 to make it easier to compare.
2: Compare the tenths place. Both numbers have 7 in the tenths place, so we move to the next position.
3: Compare the hundredths place. 0.72 has a 2 in the hundredths place, whereas 0.701 has a 0. Since 2 is greater than 0, 0.72 is greater than 0.701.
4: The thousandths place comparison is unnecessary in this context because the hundredths place already determined the greater value.
-
Compare the decimal numbers 0.392, 0.398 and 0.39.
HintsCheck each decimal place starting from the tenths to the thousandths.
Where do you find the first difference among the digits?
Consider the significance of the thousandths place in deciding the largest number when the tenths and hundredths are the same for all numbers.
You could add a zero to the end of 0.39 to make it 0.390, which helps in directly comparing it with 0.392 and 0.398 in terms of the thousandths place.
SolutionIn order from smallest to largest, the numbers are 0.39, 0.392, 0.398.
Look at the Tenths Place: All of the numbers have 3 in the tenths place, so they are the same here.
Check the Hundredths Place: They all have 9 in the hundredths place, so we still can't decide which is bigger.
Move to the Thousandths Place: Here we see the difference. 0.39 has a 0 in the thousandths place, 0.392 has 2 and 0.398 has 8.
Find the Biggest and Smallest: Since 0 is less than 2 and 2 is less than 8, we know 0.39 is the smallest, 0.392 is in the middle and 0.398 is the biggest.
-
Which glass has more juice?
HintsCompare the numbers from left to right until you find a digit that differs.
Compare the thousandths place.
Does the digit in glass A or glass B have a higher value?
Focus on the last digit of each decimal (the digit in the thousandths place).
SolutionGlass B has more juice.
Compare the Tenths Place: Look at the tenths digit of each decimal. Both 0.256 and 0.259 have 2 in the tenths place.
Compare the Hundredths Place: Both decimals have 5 in the hundredths place, so we still need to find a difference.
Compare the Thousandths Place: The thousandths place shows 6 for glass A and 9 for glass B.
Determine the Larger Amount: Since 9 is greater than 6, glass B has more juice.
-
Apply your knowledge of comparing place value to compare numbers between zero and one.
HintsLook at the last digit (thousandths place) of each decimal rate to determine which one is highest, as the tenths and hundredths places are the same for all options.
Remember, even a small difference in the rightmost decimal place can determine which option allows for a greater flow rate.
SolutionThe setting with 0.627 litres per minute will provide the most water flow over one minute.
Identify Decimal Places: Look at each number to recognise the digits in the tenths, hundredths and thousandths places.
Compare the Tenths Place: All three settings have 6 in the tenths place, so no difference here.
Compare the Hundredths Place: The hundredths digits are 2 for 0.622, 2 for 0.625 and 2 for 0.627, so they are the same up to this point.
Compare the Thousandths Place: 0.622 has 2, 0.625 has 5 and 0.627 has 7. The setting with 7 in the thousandths place, which is 0.627, provides the most flow rate as it is the highest number in the smallest place value compared.
 Do you want to learn faster and more easily?
Do you want to learn faster and more easily?