Understanding the Concept of a Fraction as a Number on the Number Line
- Fractions on a Number Line
- Fractions on a Number Line – Explanation
- Fractions on a Number Line – Example
- Mixed Numbers on a Number Line
Learning text on the topic Understanding the Concept of a Fraction as a Number on the Number Line
Fractions on a Number Line
Fractions are a way to represent parts of a whole. They are used in everyday life, such as in cooking, dividing pizza among friends, and measuring distances. Knowing how to compare fractions is an important skill when managing finances, optimising recipes, or determining the fairest way to share resources among multiple people.
A fraction represents a part of a whole or, more formally, a division of any quantity into equal parts. When we place fractions on a number line, we illustrate their value by showing where they fall between whole numbers, aiding in visualising and comparing their sizes.
Fractions on a Number Line – Explanation
Placing fractions on a number line helps us visualise their magnitude relative to whole numbers and other fractions. Here’s how to understand this representation:
| Step | Description | Example | Illustration Request |
|---|---|---|---|
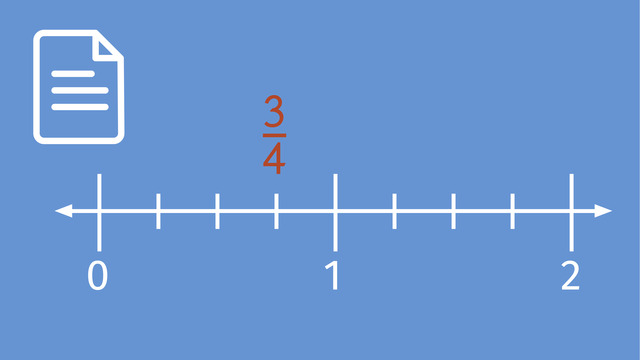
| 1 | Identify the whole numbers that the fraction lies between. | For $\frac{3}{4}$, the whole numbers are 0 and 1. |  |
| 2 | Divide the segment between these whole numbers based on the denominator of the fraction. | Divide the segment from 0 to 1 into 4 equal parts for $\frac{3}{4}$. |  |
| 3 | Place the fraction on the correct spot over the line segment according to its numerator. | Place $\frac{3}{4}$ three parts away from 0, towards 1. |  |
Fractions on a Number Line – Example
Let’s work through the process of placing the fraction $\frac{2}{3}$ on a number line.
Mixed Numbers on a Number Line
A mixed number combines a whole number and a fraction. Placing mixed numbers on a number line follows a similar process:
- Locate the whole number part on the number line.
- Add the fractional part as if starting a new fraction from the whole number identified.
Let’s work through the process of placing the fraction $2 \frac{1}{4}$ on a number line.
Fractions on a Number Line – Practice
Fractions on a Number Line – Summary
Key Learnings from this Text:
- A fraction on a number line shows its value relative to whole numbers.
- Dividing the space between whole numbers into equal parts helps in accurately placing fractions.
- Mixed numbers can be placed by locating the whole number and adding the fractional distance from it.
- Being able to place fractions on a number line can help us compare them.
 Do you want to learn faster and more easily?
Do you want to learn faster and more easily?