Multiplying Two-Digit Numbers by Two-Digit Numbers Using an Area Model—Let's Practise
- Understanding Multiplying Two-Digit Numbers by Two-Digit Numbers Using an Area Model – Introduction
- What Is Multiplying Two-Digit Numbers by Two-Digit Numbers Using an Area Model?
- Solving Multiplication Problems Using an Area Model – Examples
- Understanding Multiplying Two-Digit Numbers by Two-Digit Numbers Using an Area Model – Guided Practice
- Using Multiplying Two-Digit Numbers by Two-Digit Numbers Using an Area Model – Application
- Solving Multiplication Problems Using an Area Model – Summary
- Solving Multiplication Problems Using an Area Model – Frequently Asked Questions

Basics on the topic Multiplying Two-Digit Numbers by Two-Digit Numbers Using an Area Model—Let's Practise
Understanding Multiplying Two-Digit Numbers by Two-Digit Numbers Using an Area Model – Introduction
We're diving into the world of multiplying two-digit numbers by two-digit numbers using area models. This method helps us visualise multiplication and break it down into simpler steps. Get ready because we're going to practise this together!
What Is Multiplying Two-Digit Numbers by Two-Digit Numbers Using an Area Model?
Multiplying two-digit numbers by two-digit numbers using area models involves breaking down the multiplication problem into smaller, more manageable parts and using an area model to visualise the process. This method helps us see how the numbers are multiplied and combined.
Here are the steps to tackle multiplication using an area model:
| Step # | Action |
|---|---|
| 1 | Set up the area model by labelling the top and left side with each factor written in expanded form. |
| 2 | Multiply each part separately. |
| 3 | Add the partial products together. |
| 4 | Check your answer to ensure it makes sense with the problem. |
Let's practise understanding this method with a few examples.
Solving Multiplication Problems Using an Area Model – Examples
Example 1:
Problem: Use an area model to solve $ 51 × 79$ .
Steps to Solve the Problem:
| Step # | Action | Description |
|---|---|---|
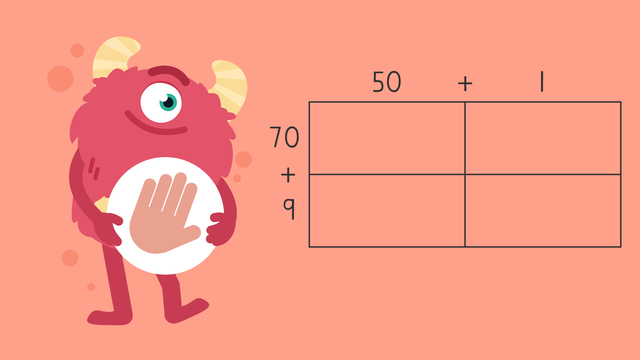
| 1 | Set up the area model by labelling the top and left side with each factor written in expanded form. | 50 + 1 and 70 + 9 |
| 2 | Multiply $ 50 × 70$ . | 3,500 |
| 3 | Multiply $ 50 × 9$ . | 450 |
| 4 | Multiply $ 1 × 70$ . | 70 |
| 5 | Multiply $ 1 × 9$ . | 9 |
| 6 | Add the partial products. | 3,500 + 450 + 70 + 9 |
| 7 | Combine the results. | 4,029 |
Solution: The product is 4,029.

Example 2:
Problem: Use an area model to solve $ 23 × 86$ .
Steps to Solve the Problem:
| Step # | Action | Description |
|---|---|---|
| 1 | Set up the area model by labelling the top and left side with each factor written in expanded form. | 20 + 3 and 80 + 6 |
| 2 | Multiply $ 20 × 80$ . | 1,600 |
| 3 | Multiply $ 20 × 6$ . | 120 |
| 4 | Multiply $ 3 × 80$ . | 240 |
| 5 | Multiply $ 3 × 6$ . | 18 |
| 6 | Add the partial products. | 1,600 + 120 + 240 + 18 |
| 7 | Combine the results. | 1,978 |
Solution: The product is 1,978.

Understanding Multiplying Two-Digit Numbers by Two-Digit Numbers Using an Area Model – Guided Practice
Take a look at these numbers: Use an area model to solve $ 34 × 52$ .
In number sentence form: 34 × 52 = 1,768
Using Multiplying Two-Digit Numbers by Two-Digit Numbers Using an Area Model – Application
Now it's your turn. Solve these problems on your own.
Use an area model to solve $ 45 × 67$ .
In number sentence form: 45 × 67 = 3,015
Solving Multiplication Problems Using an Area Model – Summary
Key Learnings from this Text:
- Solving problems using an area model can be achieved by following these steps:
| Step # | Action |
|---|---|
| 1 | Set up the area model by labelling the top and left side with each factor written in expanded form. |
| 2 | Multiply each part separately. |
| 3 | Add the partial products together. |
| 4 | Check your answer to ensure it makes sense with the problem. |
- Mastering the use of an area model for multiplying two-digit numbers is an important foundational maths skill.
Keep practising these steps, and you'll become a pro at using an area model to multiply two-digit numbers! Check out more fun maths challenges and exercises on our website to continue sharpening your skills.
Solving Multiplication Problems Using an Area Model – Frequently Asked Questions
Transcript Multiplying Two-Digit Numbers by Two-Digit Numbers Using an Area Model—Let's Practise
Razzi says get these items ready because today we're going to practise multiplying two-digit numbers by two-digit numbers using an area model. It's time to begin! Use an area model to solve fifty-one times seventy-nine. Pause the video to work on the problem and press play when you are ready to see the solution! First, set up the area model by labelling the top and left side with each factor written in expanded form. Then multiply, fifty times seventy which equals three thousand five hundred, fifty times nine which equals four hundred and fifty, one times seventy which equals seventy, and one times nine which equals nine. Then, add the partial products. Did you also get four thousand and twenty-nine? Let's tackle the next problem! Use an area model to solve twenty-three times eighty-six. Pause the video to work on the problem and press play when you are ready to see the solution! First, set up the area model by labelling the top and left side with each factor written in expanded form. Then multiply, twenty times eighty which equals one thousand six hundred, twenty times six which equals one hundred and twenty, three times eighty which equals two hundred and forty, and three times six which equals eighteen. Then, add the partial products. Did you also get one thousand nine hundred and seventy-eight? Razzi had so much fun practising with you today! See you next time!
Multiplying Two-Digit Numbers by Two-Digit Numbers Using an Area Model—Let's Practise exercise
-
Which option is the correct expanded form of 97?
HintsRemember, expanded form will show each digit expanded into its actual value.
Look at this example of the number 23 written in expanded form.
SolutionThe correct way to write the number 97 in expanded form is 90 + 7. To do that, we separated the number into the tens digit and the ones digit.
-
Solve 24 x 12.
HintsRemember the steps to solve the problem.
1) Set up the area model by labelling the top and left side with each factor written in expanded form.
2) Multiply each square in the area model.
3) Add the products together.
Here is the correct area model for this problem to help.
SolutionThe correct answer is 288.
In this image, we see all of the multiplication problems done correctly. Then, once we add up all the answers (200 + 40 + 40 + 8), we get the correct answer.
-
Solve 86 x 54.
HintsRemember the steps to solve the problem.
1) Set up the area model by labelling the top and left side with each factor written in expanded form.
2) Multiply each square in the area model.
3) Add the products together.
Here is the correct area model for this problem to help.
SolutionThe correct answer is 4,644.
In this image, we see all of the multiplication problems done correctly. Then, once we add up all the partial products(4,000 + 300 + 320 + 24), we get the correct answer.
-
Solve 79 x 67.
HintsRemember the steps to solve the problem.
1) Set up the area model by labelling the top and left side with each factor written in expanded form.
2) Multiply each square in the area model.
3) Add the products together.
Here is the correct area model for this problem.
SolutionThe correct answer is 5,293.
Here we see all of the multiplication problems done correctly. Then, once we add up all the partial products (4,200 + 540 + 490 + 63), we get the correct answer.
-
Solve 45 x 21.
HintsRemember the steps to solve the problem.
1) Set up the area model by labelling the top and left side with each factor written in expanded form.
2) Multiply each square in the area model.
3) Add the products together.
Your area model should look like the image.
SolutionThe correct answer is 945.
In this image, we see all of the multiplication problems done correctly. Then, once we add up all the answers (800 + 100 + 40 + 5), we get the correct answer.
-
What is 98 x 86?
HintsRemember the steps to solve the problem.
1) Set up the area model by labelling the top and left side with each factor written in expanded form.
2) Multiply each square in the area model.
3) Add the products together.
The first step of the area problem has been set up correctly in this image.
SolutionThe correct answer is 8,428.
In this image, we see all of the multiplication problems done correctly. Then, once we add up all the partial products (7,200 + 640 + 540 + 48), we get the correct answer.
 Do you want to learn faster and more easily?
Do you want to learn faster and more easily?