Interior and Exterior Angles of a Triangle
Do you want to learn faster and more easily?
Learning text on the topic Interior and Exterior Angles of a Triangle
Interior and Exterior Angles of a Triangle – Definition
Every triangle you see, whether it's a sign, part of a bridge, or a piece of art, embodies a fascinating aspect of geometry through its interior and exterior angles. These angles aren't just numbers; they are fundamental to understanding the triangle's shape and how it relates to the space around it. In the world of triangles, the angles inside (interior) and those formed by extending a side (exterior) play crucial roles in geometry, influencing everything from architectural designs to the essence of artistic compositions.
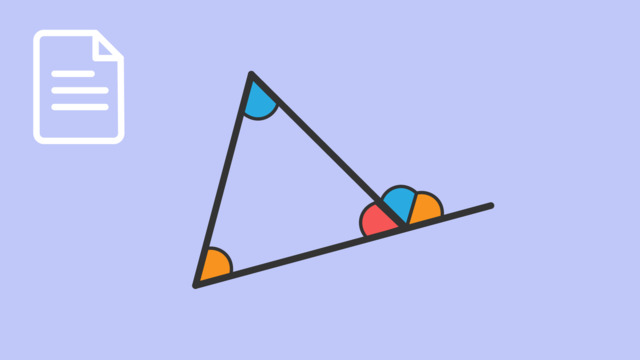
The interior angles of a triangle are the angles inside the triangle's corners. The exterior angles are formed by extending one side of the triangle and measuring the angle outside the triangle adjacent to an interior angle. The sum of the interior and exterior angles should always be 180 degrees.
Angle Sum Theorem
The Angle Sum Theorem (also known as the Triangle Sum Theorem) tells us that no matter what type of triangle we have, the three interior angles always add up to 180 degrees.
The ∠ symbol means angle. It is usually followed by a letter to describe which angle it is talking about. For example, here ∠A means angle A.
Let’s take a look at some problems to solve by applying the Angle Sum Theorem. Is it possible to have a triangle with angle measurements of $45^\circ$, $15^\circ$, and $40^\circ$?
- Find the sum of the given angles, $45 + 15 + 40 = 100$.
- The angle sum does not equal exactly $180$ degrees, and therefore these angle measurements are impossible for a triangle.
Is it possible to have a triangle with angle measurements of $55^\circ$, $20^\circ$, and $105^\circ$?
- Find the sum of the given angles, $55 + 20 + 105 = 180$.
- The angle sum is equal to exactly $180$ degrees, so, therefore these angle measurements are possible for a triangle.
Go ahead and apply the Angle Sum Theorem in different scenarios:
Finding Missing Angles using the Angle Sum Theorem
Now that you understand that the angle sum theorem states that a triangle’s angles must have a sum of exactly $180$ degrees, let’s apply this knowledge to find missing angles, without needing to use a protractor.
What is the measurement of the missing angle?
- 1️⃣The sum of the interior angles must equal exactly $180^\circ$, and since we are missing one angle measurement, this value can be represented with a variable, $x$.
- 2️⃣The equation $55 + 70 + x = 180$ can be used to represent the interior angles of this triangle.
- 3️⃣Solve the $x$ to find the missing angle.
$55+70+x=180$
$125+x=180$
$x=55$
Try it yourself:
Exterior Angle Theorem
The Exterior Angle Theorem states that the measure of an exterior angle of a triangle is equal to the sum of the measures of the two non-adjacent interior angles (also referred to as opposite interior angles).
Think of the Exterior Angle Theorem as opening a door slightly. That opening is the exterior angle of a triangle, similar to the part of the door still closed, which represents the two non-adjacent interior angles. Essentially, the open part (exterior angle) matches the closed part's width (sum of the two interior angles). It shows that the exterior angle fills in the space the other angles don't, making sure all angles at that vertex add up to a straight line or $180$ degrees.
Applying the Exterior Angle Theorem
Let's explore how the Exterior Angle Theorem works with a few examples.
If one interior angle of a triangle is $60^\circ$ and another is $70^\circ$, what is the measure of the exterior angle adjacent to these angles?
- 1️⃣ First, find the sum of the interior angles: $60 + 70 = 130^\circ$.
- 2️⃣ According to the Exterior Angle Theorem, the exterior angle is equal to the sum of these two interior angles, so the exterior angle is $130^\circ$.
If a triangle has interior angles of $52^\circ$ and $58^\circ$, what is the measure of the exterior angle next to these angles?
- 1️⃣ Add the interior angles: $52 + 58 = 110^\circ$.
- 2️⃣ The exterior angle will be $110^\circ$, as it equals the sum of the non-adjacent interior angles.
Go ahead and apply the Exterior Angle Sum Theorem in different scenarios:
Interior and Exterior Angles of a Triangle – Problem Solving
Use your knowledge of the interior and exterior angles of a triangle to find the missing angle measurements in the problems. Being familiar with finding angles using equations will help solve these problems more easily!
For more practice problem-solving with angles, check out What are Supplementary and Complementary Angles? and Conditions for a unique triangle to learn more!
Interior and Exterior Angles of a Triangle – Summary
Key Learnings from this Text:
- The Angle Sum Theorem confirms that every triangle's interior angles sum up to 180 degrees.
- The Exterior Angle Theorem states that an exterior angle of a triangle is equal to the sum of the two non-adjacent interior angles.
- Understanding these principles, including the Angle Sum Theorem and Exterior Angle Theorem, is crucial for solving various geometric problems.
Interior and Exterior Angles of a Triangle – Frequently Asked Questions
Interior and Exterior Angles of a Triangle exercise
-
Which statement about the angles of a triangle is correct?
HintsAll triangles have three interior angles.
Angles $a+b+c=180^\circ$.
SolutionThe sum of the interior angles in a triangle is always equal to $180$ degrees.
-
What is the measurement of angle $a$?
HintsRemember, the interior angles of a triangle have a sum of 180 degrees.
If you know two of the three angles, you can either
- subtract the known angles from $180$, or
- add the known angles together, and subtract from $180$
Let's look at an example:
To find $x$, subtract the known angles from $180$.
$180-40-70=70^\circ$
$x=70^\circ$
Solution$a=80^\circ$
Two angles are known $40^\circ$ and $60^\circ$.
Subtract these known values from $180$.
$180-40-60=80^\circ$
-
Find the missing angle.
HintsThe measure of an exterior angle of a triangle is equal to the sum of the measures of the two non-adjacent interior angles.
The square seen in one angle means that that angle is $90^\circ$, which is also known as a right angle.
The two interior remote angles in this example are $30^\circ$ and $90^\circ$.
SolutionThe missing angle measurement is $120^\circ$.
The sum of the two remote interior angles is equal to the exterior angle.
$30+90=120$
-
Apply your understanding of the relationships of interior and exterior angles.
HintsThe interior angles of a triangle always add up to 180 degrees.
The measure of an exterior angle of a triangle is equal to the sum of the measures of the two non-adjacent interior angles.
Number 3 in this question has two correct equations for the answers.
SolutionThe value of angle $x$ is $\bf{25^\circ}$.
The value of angle $y$ is: $\bf{160^\circ}$.
Angle $z$ can be found by using the equations $\bf{102+z=180}$ or $\bf{44 + 34 = z}$.
-
Identify interior and exterior angles of a triangle with extended sides.
HintsInterior angles are found inside the triangle.
Exterior angles are created when the triangle's legs are extended.
Triangles always have 3 interior angles and they have a sum of 180$^\circ$.
SolutionThe triangle has 3 interior angles as you can see labelled.
Three of the six exterior angles are also labelled.
-
Demonstrate your understanding of interior and exterior triangle angle measurements.
HintsThe sum of the interior angles of a triangle is always 180 degrees.
The measure of an exterior angle of a triangle is equal to the sum of the measures of the two non-adjacent interior angles.
To find the measure of angle $a$, notice that the angle adjacent is equal to $105^\circ$. Since angles $a$ and $105^\circ$ are on a straight line, the sum is $180^\circ$.
SolutionMissing Angle Measurements:
- $a=75^\circ$
- $b=105^\circ$
- $c=165^\circ$
- $x=60^\circ$
- $y=15^\circ$
- $z=120^\circ$
