Angles in Shapes
Basics on the topic Angles in Shapes
Angles of Polygons - Introduction
Angles in two-dimensional shapes, such as polygons, are an essential aspect of geometry that lay the foundation for many mathematical and real-world applications. This article explores the basics of angles in 2D shapes, their properties, calculations and much more.
Basics of Polygons – Definitions
Let's talk about polygons. They come in two main types: regular polygons and irregular polygons. Polygons are shapes with straight sides. They can be regular (where everything's the same) or irregular (where everything can be different).
Regular Polygons: Imagine a shape where all the sides are the same length, and all the angles are the same size. That's a regular polygon! These shapes are like perfect team players; everything matches and fits together nicely. If you've ever seen a stop sign, you've seen a regular polygon. It's a perfect fit in many puzzles, designs and games!
Irregular Polygons: Now, think of a shape where the sides and angles don't match. That's an irregular polygon! These shapes are like wild adventurers; no two sides or angles need to be the same. They're really useful for drawing things that you find in nature, like mountains or rivers.
Whether you're drawing, building with blocks or solving fun puzzles, understanding polygons helps you see the world in a whole new way!
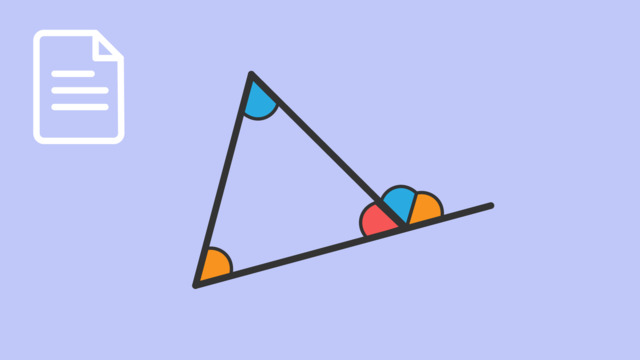
Interior and Exterior Angles in Shapes
There are two types of angles that you can find in shapes.
Interior angles are the angles found inside a polygon. They vary depending on the number of sides.
Exterior angles are the angles formed outside the polygon by extending one side of the polygon. For any polygon, the sum of exterior angles is always 360 degrees.
We will explore these angles in more detail shortly.
Exploring the Interior Angles of a Polygon
Have you ever wondered how the angles inside different shapes add up? Let's explore!
Triangles: Triangles are like a slice of pie with three sides, and the angles inside always add up to 180 degrees. No matter how you change the shape of the triangle, the angles inside will always make a straight line if you put them together.
Quadrilaterals: Quadrilaterals have four sides, like a piece of paper or a television screen. If you add up all the corners' angles, you will get 360 degrees, just like a full turn! We can also call these interior angles right angles in shapes.
Why is this useful to know? Well, the total sum of interior angles in different shapes helps us solve puzzles and mysteries. For example, if you’re looking for missing angles in shapes and you know two angles in a triangle, you can find the third one just by subtracting the angles you know from 180!
Similarly, if you know three angles in a quadrilateral, you can find the missing angle by subtracting the known angles from 360.
Exploring the Exterior Angles of a Shape
Have you ever looked at the angles outside a shape like a triangle or a star? These outside angles are called exterior angles, and they have some surprising secrets!
Exterior Angles: These are the angles on the outside edges of shapes, and they're like the little twists or turns between the sides. They're essential in all kinds of different shapes, and they can help us understand how the shape bends and moves.
So why do these outside angles matter? Imagine you have a paper cut-out of a shape, and you want to fold it into a 3D object. Understanding how these angles work helps you figure out how to fold it properly. It's like a map to folding shapes!
While there is some remembering to do when figuring out interior angles of different shapes, exterior angles always add up to 360 degrees, regardless of the shape!
Common Polygons and Their Angles
Let's talk about some of the commonly found shapes like triangles, squares, pentagons and hexagons and find out more about polygon interior angles. Did you know that they have angles that add up to special numbers? It's like they have their own angles in shapes rules, and it's so much fun to find out what they are! Below we can find out about sum of interior angles of a polygon for different 2D shapes.
Triangles: These three-sided shapes have angles that add up to 180 degrees.
Quadrilaterals: These are like squares or rectangles, and their angles add up to 360 degrees.
Pentagons: With five sides, pentagons have angles that add up to 540 degrees.
Hexagons: Have you ever looked at a beehive's honeycomb? Those are hexagons, and their angles add up to 720 degrees!
And guess what? If a shape has sides that are all the same length, like a perfect square, the angles behave in a special way called congruent. That means they're all the same.
| Shape | Number of Sides and Angles | Total Sum of Interior Angles | Total Sum of Exterior Angles |
|---|---|---|---|
| Triangle | 3 | 180 degrees | 360 degrees |
| Quadrilateral | 4 | 360 degrees | 360 degrees |
| Pentagon | 5 | 540 degrees | 360 degrees |
| Hexagon | 6 | 720 degrees | 360 degrees |
Understanding these interior angles in shapes rules is like knowing a shape's hidden language. Whether you're drawing pictures, building something or just looking at the world around you, knowing these angle secrets makes everything more exciting! So next time you see one of these shapes, you'll know a little more about what makes it special.
Angles of Polygons – Summary
Polygons are 2D shapes categorised as regular (with congruent sides and angles) or irregular. They have interior angles whose sum varies by shape: triangles 180°, quadrilaterals 360°. Exterior angles in all polygons sum up to 360°. Polygons with equal sides also have equal angles. Understanding these angles is fundamental for geometry and real-world applications.
Have you enjoyed finding out about interior angles of a polygon? If you think you know how to work out interior angles and how to find interior angles of polygons, have a look at our angles in shapes worksheet, interactive exercises and activities.
Frequently Asked Questions about Angles of Polygons
Transcript Angles in Shapes
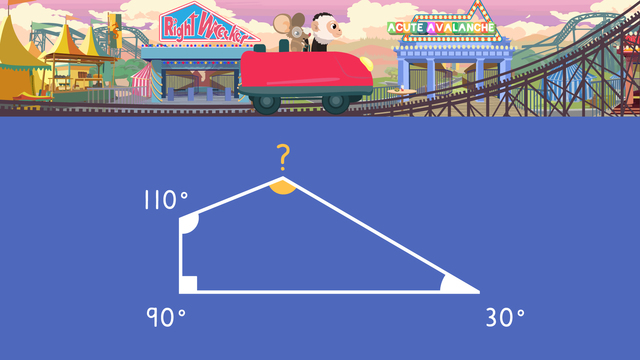
“The park is closing soon and we have time for one more ride, Nia.” “Let’s go on one of the roller coasters!” "Hmmm, which one?" “ I want to go on the one with the biggest drop!” We can help Nico and Nia work out which ride to choose by looking at the inside angles created by the roller coaster rides. "Angles in Shapes." All geometric shapes have angles based on their number of sides. A triangle is made up of three angles and when we add up their measurements, it will always equal one hundred and eighty degrees. Quadrilaterals have four angles and they will always total three hundred and sixty degrees. When we look at the outlines of the roller coaster rides, we can see that they make geometric shapes. We can use what we know about angle measurements in shapes to find the measurement of the missing angle at the top of each ride. Let's look at the Obtuse Oblivion. What do you observe about the shape of this ride? The ride is shaped like a triangle and it has an obtuse angle at the top. One angle measures forty degrees, and one measures thirty degrees. Let’s think about what we know. We know the measure of two angles and the total triangle will measure one hundred and eighty degrees. First, solve this by adding the known angles, then subtracting the sum from one hundred and eighty. Forty plus thirty equals seventy. One hundred and eighty minus seventy equals one hundred and ten. The missing angle measures one hundred and ten degrees. Next, we’ll check out the Right Wrecker! This one looks intense! What do you notice about the shape and angles created by this ride? This shape is a quadrilateral, which means there are four angles and they measure three hundred and sixty degrees in total. We are given the angle measurements for three of the angles. We have one hundred and ten degrees and thirty degrees. Even though there isn’t a number here, from the box, we know this one is a right angle. What is the measurement of a right angle? It is ninety degrees. To solve for this missing angle, add the known angles and subtract the sum from three hundred and sixty. First, we'll add one hundred and ten, ninety and thirty. What is the sum of these angles? The sum of these angles is two hundred and thirty degrees. Now, subtract the sum from three hundred and sixty. What is the measure of the missing angle? One hundred and thirty degrees. One more ride to consider the Acute Avalanche! This coaster is made up of two shapes. Let’s split them apart and solve for the missing angles in each. What is this first shape? This is a parallelogram, which is a type of quadrilateral. We can solve for the missing angle using what we know about parallelograms. A parallelogram has two sets of opposite angles of equal measure. If this angle is one hundred and twenty degrees, then this angle is also one hundred and twenty degrees. If this angle is sixty degrees what is the measurement of this one? The opposite angle would also be sixty degrees. To check our answer, add one hundred and twenty, sixty, one hundred and twenty, and sixty. These angles total three hundred and sixty degrees. Let's look at the equilateral triangle. What do you notice about the angles? We aren’t given any of the measurements, but we see they are all the same size. Since a triangle always has a total of one hundred and eighty degrees, we can use that to work out the measurements of the angles. What number can we add three times to equal one hundred and eighty degrees? Sixty! Sixty plus sixty plus sixty equals one hundred and eighty. Remember, geometric shapes have angles that have set totals. We can solve for inside angle measurements in a few ways. One way is to find the sum of angles given and subtract that number from total degrees in a given shape. Or use what you know about a shape to find the missing angle measurements. “I think Acute Avalanche looks like it has the best drop!” “Let’s go!” "Nico, are we there yet?" "Yes, we're at the top!" "Hang on!" "Woo hoo!"
Angles in Shapes exercise
-
What are the missing angles?
HintsRemember, this box in the bottom right corner means there is a right-angle, which is always 90°.
Remember, all of the internal angles of a triangle equal 180°.
Remember, all of the internal angles of a quadrilateral equal 360°.
Remember, opposite angles in parallelograms are equal.
SolutionHere are the missing angles:
- The parallelogram had two missing angles of 75° each.
- The triangle had one missing angle of 90° and one missing angle of 65°.
Parallelogram
- The first part of the roller coaster was made of a parallelogram.
- We know that parallelograms are quadrilaterals, so opposite angles are equal and all internal angles add up to 360°.
- We are given two angles of 105°. 105 + 105 = 210.
- We then subtract 210 from 360, which equals 150.
- We then divide 150 by 2, to get 75.
- Therefore each of the missing angles in the parallelogram are 75°.
- We know that all internal angles of a triangle add up to 180°.
- We are given one angle of 25°.
- We can then see a square box in the corner of the triangle meaning it is a right-angle. Right-angles always equal 90°.
- We then add 25 + 90 = 115.
- We then subtract 115 from 180, which equals 65.
- The missing angle is 65°.
-
What are the missing angles?
HintsRemember, the internal angles of a triangle add up to 180°.
Remember, the internal angles of a quadrilateral add up to 360°.
Remember, in a square or parallelogram, opposite angles are equal.
SolutionHere are the missing angles for each shape:
Square
- A square is a quadrilateral, therefore all internal angles must add up to 360°.
- All internal angles of a square are also right-angles, meaning that the missing angle must be 90°.
- 90° + 90° + 90° + 90° = 360°.
- All angles are different in a scalene triangle but all internal angles still add up to 180°.
- 80° + 40° = 120°.
- 180° - 120° = 60°.
- The missing angle is therefore 60°.
- A parallelogram is a quadrilateral, therefore all internal angles add up to 360°.
- We also know that opposite angles are equal.
- 60° + 60° = 120°.
- 360° - 120° = 240°.
- 240° $\div$ 2 = 120°.
- The missing angle is therefore 120°.
- An isosceles triangle has two equal angles and all internal angles add up to 180°.
- We were given the two equal angles of 80°.
- 80° + 80° = 160°
- 180° - 160° = 20°
- The missing angle is 20°.
-
Find the missing angles.
HintsHow do you spot a right-angle? Remember, all right-angles are 90°.
Add up the angles given in the triangles and parallelograms and work out what is missing.
Remember:
- all internal angles in a triangle add up to 180°.
- all internal angles in a parallelogram (a quadrilateral) add up to 360°.
SolutionHere are the correctly highlighted angles:
Ladder
- The shape made here is a rectangle.
- All angles are right-angles, so are all 90° and are highlighted in green.
- Here is an equilateral triangle.
- 60° + 60° = 120°
- 180° - 120° = 60°
- The missing angle is 60° and is highlighted in blue.
- Here is another equilateral triangle.
- 60° + 60° = 120°
- 180° - 120° = 60°
- The missing angle is 60° and is highlighted in blue.
- The shape here is a rectangle.
- All angles are right-angles so are all 90° and are highlighted in green.
- Opposite angles are equal in a parallelogram.
- 150° + 150° = 300°.
- 360° - 300° = 60°.
- 60° $\div$ 2 = 30°
- The missing angles are therefore both 30° and are highlighted in yellow.
- Here we have a right-angled triangle.
- One missing angle is a right-angle and is 90° so is highlighted in green.
- 60° + 90° = 150°.
- 180° - 150° = 30°.
- The top angle is therefore 30° and is highlighted in yellow.
-
Can you spot the mistakes?
HintsDo any of the shapes have right-angles?
Remember, in parallelograms:
- All internal angles add up to 360°
- Opposite angles are equal
All internal angles in a triangle add up to 180°.
Look at the triangle with the angles 62°, 48° and 70°.
Add 62 + 48 + 70 = 180.
The internal angles of a triangles always equal 180° so this one is correct so we don't highlight it.
Use this method to work through the other shapes.
SolutionHere are the angles that should have been highlighted:
- In the scalene triangle we were given two angles of 48° and 62°. 48 + 62 = 110. 180 - 110 = 70 so the angle was 70° and this is correct.
- In the parallelogram we were given two angles of 125°. 125 + 125 = 250. 360 - 250 = 110. 110 $\div$ 2 = 55 so the angles should have been 55°. The two 45° angles are incorrect.
- In the isosceles triangle we were given two angles of 73°. 73 + 73 = 146. 180 - 146 = 34 so the angle is 34°. 33° is incorrect.
- In the right-angled triangle we were given an angle of 15° and a right-angle which is 90°. 90 + 15 = 105. 180 - 105 = 75 so the angle is 75°. 65° is incorrect.
-
What is the missing angle?
HintsCan you remember what all internal angles of a triangle add up to?
The internal angles of a triangle always add up to 180°.
If you add the angles given, what is the difference between that and 180°?
SolutionThe missing angle was 35°.
- All internal angles of a triangle add up to 180°.
- 80 + 65 = 145.
- 180 - 145 = 35.
- Therefore the missing angle is 35°.
-
What are the angles that Nico and Nia saw?
HintsA right-angle is always 90°.
The internal angles of a quadrilateral add up to 360°.
The internal angles of a triangle add up to 180°.
An isosceles triangle has two equal angles.
SolutionThe first roller coaster that Nia and Nico saw had a triangle shape in it. They measured two angles of 72° and 41° so the final angle must be 67°.
- 72 + 41 = 113.
- 180 - 113 = 67
- The missing angle is therefore 67°.
- A parallelogram is a quadrilateral so all inside angles add up to 360°.
- 84 + 84 = 168
- 360 - 168 = 192
- 192 $\div$ 2 = 96
- The missing angles are therefore 96° each.
- One angle is a right angle so is therefore 90°.
- 90 + 29 = 119
- 180 - 119 = 61
- Therefore the missing angle is 61°.
- Isosceles triangles have two equal angles.
- 180 - 18 = 162
- 162 $\div$ 2 = 81
- Therefore the missing angles are 81° each.
 Do you want to learn faster and more easily?
Do you want to learn faster and more easily?