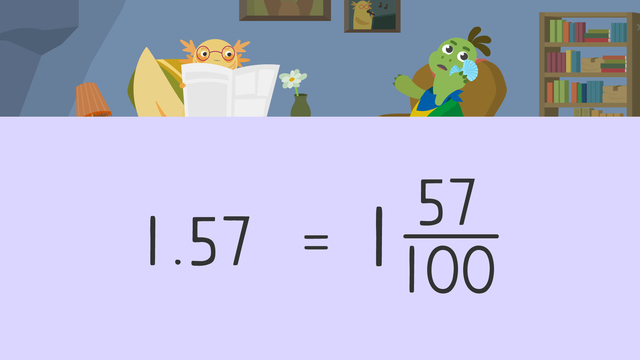Decimals Greater than 1 as Fractions — Let's Practice!
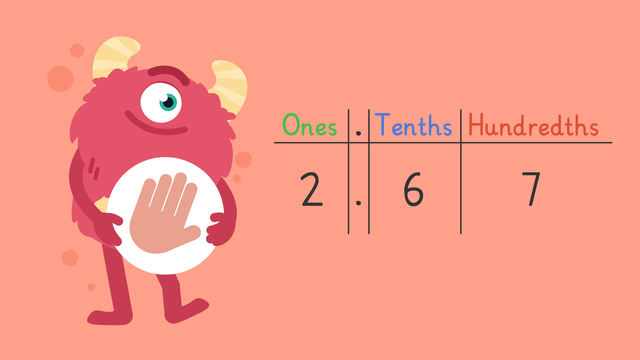
Basics on the topic Decimals Greater than 1 as Fractions — Let's Practice!
How do we convert decimals greater than one to fractions? Watch this video to learn about converting numerators and making decimals into fractions.
Transcript Decimals Greater than 1 as Fractions — Let's Practice!
Razzi says get these items ready because today we're going to practise writing decimals greater than one as fractions. It's time to begin! Write two point six seven as a fraction. Pause the video to work on the problem and press play when you are ready to see the solution! Write each place value as a fraction. Convert the fractions to have the greatest denominator, one hundred. Two ones becomes two hundred hundredths. Six tenths becomes sixty hundredths. Now add the numerators, keeping the denominator the same. Simplify to get, two and sixty-seven hundredths. Did you also get two and sixty-seven hundredths? Let's tackle the next problem! Write six point nine as a fraction. Pause the video to work on the problem and press play when you are ready to see the solution! Write each place value as a fraction. Convert the fractions to have the greatest denominator, ten. Six ones becomes sixty-tenths. Now add the numerators, keeping the denominator the same. Simplify to get, six and nine-tenths. Did you also get six and nine-tenths? Let's tackle the final problem! Write four point zero one as a fraction. Pause the video to work on the problem and press play when you are ready to see the solution! Write each place value as a fraction ignoring the zero, since it is a placeholder. Convert the fractions to have the greatest denominator, one hundred. Four ones becomes four hundred hundredths. Now add the numerators, keeping the denominator the same. Simplify to get four and one hundredth. Did you also get four and one hundredth? Razzi had so much fun practising with you today! See you next time!
Decimals Greater than 1 as Fractions — Let's Practice! exercise
-
Match the decimal to a fraction.
HintsWrite each place value as a fraction.
After you write each place value as a fraction, multiply the numerator and denominator so every denominator equals 100. Add the numerators together.
$\frac{100}{100}$ + $\frac{30}{100}$ + $\frac{8}{100}$ = $\frac{138}{100}$ = 1 $\frac{38}{100}$
SolutionWrite each place value as a fraction.
- For example: 1.67 = $\frac{1}{1}$ + $\frac{6}{10}$ + $\frac{7}{100}$
- For example: 1.67 = $\frac{100}{100}$ + $\frac{60}{100}$ + $\frac{7}{100}$
- For example: 1.67 = $\frac{167}{100}$
_____________________________
- 2.32 = 2$\frac{32}{100}$
- 3.80 = 3$\frac{8}{10}$
- 1.05 = 1$\frac{5}{100}$
-
True or False?
HintsWrite each place value as a fraction.
Multiply the fractions so they all have a common denominator. Add the numerators together.
Convert to a mixed number.
Double check place value. Are the correct digits in the tenths or hundredths places?
Solution- 2.67 = 2$\frac{67}{100}$ true
- 4.07 = 4$\frac{7}{100}$ true
- 3.2 = 3$\frac{2}{100}$ false. 3.2 = 3$\frac{2}{10}$
- 5.92 = 5$\frac{92}{10}$ false. 5.92 = 5$\frac{92}{100}$
-
Can you write decimals and fractions?
HintsNumber 1 is missing the ones place. Which number is in the ones place?
5.47 = $\frac{500}{100}$ + $\frac{40}{100}$ + $\frac{7}{100}$
Number 2 is missing the decimal. How can you convert 3$\frac{21}{100}$ to a decimal?
3$\frac{21}{100}$ = $\frac{300}{100}$ + $\frac{20}{100}$ + $\frac{1}{100}$
What is this as a decimal?
Solution- 5.47 = 5$\frac{47}{100}$
- 3.21 = 3$\frac{21}{100}$
-
Convert the fraction into a decimal.
HintsWork out which place value each digit is in.
In 2$\frac{6}{10}$, the 2 is in the ones place and the 6 is in the tens place.
As an example, 1$\frac{6}{10}$ converts to 1.6.
Solution2$\frac{6}{10}$ = 2.6
5$\frac{8}{10}$ = 5.8
4$\frac{4}{100}$ = 4.04
9$\frac{73}{10}$ = 9.73 -
Match the fraction to the correct decimal.
HintsWrite each place value as a fraction. Make sure to check the tenths and hundredths place.
The 2 is in the tenths place. The 7 is in the hundredths place.
Put the parts of the mixed number into a place value chart.
Solution1 $\frac{27}{100}$ = 1.27
- There is a 1 in the ones place. $\frac{100}{100}$
- There is a 2 in the tenths place. $\frac{20}{100}$
- There is a 7 in the hundredths place. $\frac{7}{100}$
-
True or False?
HintsThere are four place values in 2.369. What are they?
Use the chart to help you. Create fractions for each place value. The next place value after hundredths is thousandths, so the fraction that represents the 9 in the thousandths place is $\frac{9}{1000}$
SolutionTrue.
2$\frac{369}{1000}$ = 2.369


 Do you want to learn faster and more easily?
Do you want to learn faster and more easily?