Decimal Tenth and Hundredth

Basics on the topic Decimal Tenth and Hundredth
Converting Decimals – Tenths and Hundredths
A decimal represents a part of a whole, like a fraction, but shows it slightly differently. A decimal fraction is a fraction with a denominator that is a power of ten, such as ten or one hundred. Next, let’s take a look at how to turn decimals to fractions.
Converting Decimals to Fractions
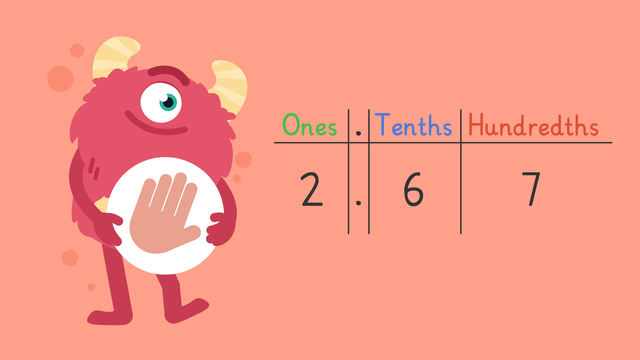
When converting decimals, ask yourself, “What is the place value of the last digit?”. If it is a tenth decimal place, then the denominator is ten. The numerator represents how many equal parts of a whole we have, which is the number you see in the tenth place in a decimal. What’s the tenth place in a decimal? Remember the tenths place is the first place value to the right of the decimal point. Let’s look at how to find tenths place in a decimal to try some converting decimals to fractions practise. Below, we see one tenth as a decimal. In order to change or convert it into a fraction ask yourself, “What is the place value of the last digit?”. If the last digit is in the tenth place in a decimal, then the denominator is ten. The numerator represents how many equal parts of a whole we have, which is the number you see in the tenth place. Zero point one is equal to 1 tenth as a fraction. Equally, 1 tenth in decimal form is zero point one.
Converting Fractions to Decimals
When converting fractions to decimals, ask yourself, “What is the place value represented in the denominator?”. If denominator is one hundred then we need to look at the hundredth column. The numerator represents how many equal parts of a whole we have, which is the number you see in the hundredth column. Remember the hundredth place is the second place value to the right of the decimal point. Let’s look at converting more fractions to decimals.
Below, we see zero point zero one. In order to change or convert it into a fraction ask yourself,“What is the place value represented in the denominator?”. If it is a hundredth decimal place, then the denominator is one hundred. The numerator represents how many equal parts of a whole we have, which is the number you see in the hundredth place. One hundredth as a fraction is equal to zero point zero one as a decimal.
Converting Decimals – Summary
When converting decimals, ask yourself, “What is the place value of the last digit?”. If it is in the tenths column, then the denominator is ten. The numerator represents how many equal parts of a whole we have, which is the number you see in the tenth column in the decimal. When converting fractions, ask yourself, “What is the place value represented in the denominator?”. If it is one hundred, then the last digit of the decimal will be in the hundredths column. The numerator represents how many equal parts of a whole we have, which is the number you see in the hundredth decimal place. The table below shows some examples of how decimals can be displayed as fractions.
| Decimal | Fraction |
|---|---|
| 0.1 | $\frac{1}{10}$ |
| 0.4 | $\frac{4}{10}$ |
| 0.01 | $\frac{1}{100}$ |
| 0.07 | $\frac{7}{100}$ |
Want some more converting fractions to decimals and converting decimals to fractions practise? You can find examples along with a converting fractions to decimals worksheet and a converting decimals to fractions worksheet on this website.
Transcript Decimal Tenth and Hundredth
"And then my Mum was like, 'don't forget your turtleneck!', can you believe that!?" "Hi, can I help you?" "I need some help converting decimals and fractions to unlock these tickets for the big show tonight. If you help me, I'll give them to you." Let's help the Tricky Troll by Converting Decimals and Fractions. A decimal number represents a part of a whole in the same way as a fraction, but is written slightly differently. We can convert decimals in to fractions and fractions in to decimals. The easiest way to convert a decimal in to a fraction is to change it into tenths or hundredths. Here is the decimal 'zero point eight' represented using a base ten block. When converting decimals into fractions we can write the decimal on a place value chart like this. What is the place value of the the last digit? We see the eight is in the TENTHS place, so the denominator is ten. As we have an EIGHT in the tenths column, this means that we have EIGHT tenths. So our numerator is eight. Now that we have eight tenths in decimal notation AND fraction notation, what do you notice about them? They are EQUIVALENT because they both represent eight parts of one whole, zero point eight is equal to eight tenths. Now let's help the Tricky Troll by converting the decimal 'zero point three five' in to a fraction. First, write the decimal on the place value chart. What is the place value of the last digit? We can see that the five is in the HUNDREDTHS column, so the denominator is one hundred. What is the numerator? We have thirty-five hundredths, or three tenths and five hundredths, so our numerator is thirty-five. Zero point three five written as a fraction is thirty-five hundredths. Finally, we have the fraction nine-hundredths. This time, we are converting a fraction into a decimal. How can we convert nine-hundredths into a decimal? Since the denominator is one hundred, we know it is representing the value, HUNDREDTHS. How many hundredths do we have? Let's use our place value chart to help. The numerator is nine, so write nine in the hundredths column. How many tenths do we have? We don't have any tenths, so we write a zero in the TENTHS column. How many ones do we have? We don't have any ones so we write a zero in the ONES column. Nine hundredths written as a decimal is zero POINT zero nine. We've converted all the decimals and fractions! While they get ready for the show, let's summarize. Remember, we can convert fractions into decimals and decimals into fractions and this is most simply done when the fraction is out of ten or one hundred. When converting decimals into fractions, check what is the place value of the last digit when written on the place value chart? The place value is your denominator, and the number written in that column is the numerator. It represents how many parts of the whole there are. When converting fractions into decimals, the denominator represents the place value, and the numerator represents how many equal parts of a whole we have. "I love Blue Troll Group!" "This is TURTALLY awesome!"
Decimal Tenth and Hundredth exercise
-
What is the equivalent fraction?
HintsBegin by writing the decimal onto a place value grid. Here, 0.8 is written onto the grid.
Identify the value of the digit by finding its position on the place value grid. An 8 in the tenths column means eight tenths.
To make a fraction that has a denominator of 100 into tenths, divide both the denominator and the numerator by 10. For example, $\frac{60}{100}$ ÷ 10 = $\frac{6}{10}$ which is the same as 0.6.
SolutionThe equivalent fractions and decimals are:
- 0.2 = $\frac{2}{10}$ = $\frac{20}{100}$
- 0.5 = $\frac{5}{10}$
- 0.6 = $\frac{6}{10}$
- 0.8 = $\frac{8}{10}$
-
How do we write fractions as decimals?
HintsThe denominator of the fraction is 100, so the numbers need to go as far as the hundredths column of the place value grid.
There are no ones in $\mathbf{\frac{75}{100}}$, so there will be no ones in the ones column of the place value grid.
Solution$\mathbf{\frac{75}{100}}$ is made up of 7 tenths and 5 hundredths, or 75 hundredths, so there will be a 7 in the tenths column and a 5 in the hundredths column.
-
Representing numbers.
HintsLook how many parts there are in total, this is the denominator. How many parts are shaded? This is the numerator.
Once you know the fraction out of ten or one hundred, write this onto a place value grid. For example, 25 hundredths would be written as 0.25.
Simplify the fraction you are trying to find, to find an equivalent fraction. For example, 0.25 is the same as $\frac{25}{100}$, so would be simplified to $\frac{1}{4}$.
Solution- The first image shows 5 parts out of 10 shown. This is the same as a half or fifty out of one hundred.
- The second image shows 75 parts shaded out of 100. This is the same as seventy-five out of one hundred or zero point seven five.
- The third image shows 30 parts out of 100 or 3 out of ten. This is the same as zero point three, thirty hundredths or three tenths.
-
Order the fractions and decimals.
HintsBegin by converting all the amounts into the same value. You could make every amount into hundredths.
To convert $\frac{7}{10}$ to hundredths, multiply the numerator and denominator by ten to get $\frac{70}{100}$.
To convert a decimal into hundredths, write the decimal onto a place value grid. For example, 0.08 would have zero ones, zero tenths and eight hundredths ($\frac{8}{100}$).
Try drawing a hundred square and shading in the amount shown in the numerator when the denominator is 100, then see which fraction has the most shaded in.
SolutionThe correct order from smallest to greatest values is:
- 0.04 or $\frac{4}{100}$. 0.04 is equivalent to zero ones, zero tenths and four hundredths.
- 0.2 or $\frac{20}{100}$. 0.2 is equivalent to zero ones and two tenths which is equivalent to twenty hundredths.
- $\mathbf{\frac{4}{10}}$ or $\frac{40}{100}$.
- 0.48 or $\frac{48}{100}$. 0.48 is equivalent to zero ones, four tenths and eight hundredths which is the same as forty-eight hundredths.
- $\mathbf{\frac{63}{100}}$
- $\mathbf{\frac{7}{10}}$ or $\frac{70}{100}$
-
Find the fraction to match.
HintsWhen we write 0.03 onto a place value grid, how many hundredths are there?
Can you find a fraction that has 100 as the denominator?
Solution0.03 = $\mathbf{\frac{3}{100}}$. When we write the decimal onto a place value grid, we can see that there are zero ones, zero tenths and three hundredeths.
-
Converting fractions to decimals.
HintsTo convert a fraction to a decimal, first convert the fraction so that it has a denominator of 10 or 100. To convert $\frac{6}{20}$, we can divide the numerator and denominator by 2 to get $\frac{3}{10}$.
Once the fraction is written as out of 10 or 100, write it onto a place value grid. $\frac{3}{10}$ has zero ones, 3 tenths and zero hundredths, so is equivalent to 0.3.
When a fraction has 5 as a denominator, multiply both the numerator and denominator by two so that the fraction is out of 10.
Solution- $\frac{35}{100}$ = 0.35 ✅
- $\frac{2}{10}$ = 0.02 ❌ $\frac{2}{10}$ = 0.2
- $\frac{3}{5}$ = 0.3 ❌ $\frac{3}{5}$ = 0.6
- $\frac{4}{5}$ = 0.8 ✅
- $\frac{12}{20}$ = 0.6 ✅
- $\frac{2}{20}$ = 0.2 ❌ $\frac{2}{20}$ = 0.1
- $\frac{7}{100}$ = 0.07 ✅
- $\frac{1}{5}$ = 0.5 ❌ $\frac{1}{5}$ = 0.2
 Do you want to learn faster and more easily?
Do you want to learn faster and more easily?


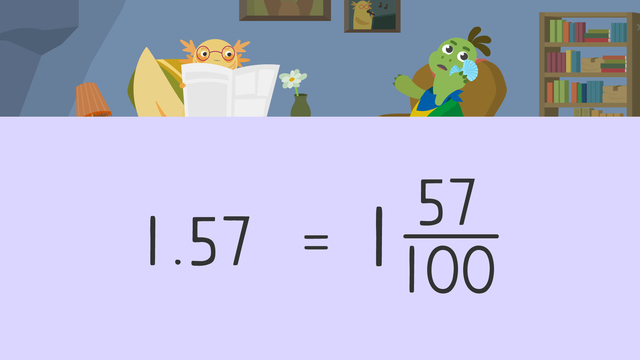









It really help and now I know