Order of Operations
Do you want to learn faster and more easily?
Learning text on the topic Order of Operations
Order of Operations – Introduction
Exploring mathematics can sometimes feel like navigating a vast ocean of numbers and equations. To journey through these waters, one essential tool is understanding the order of operations. Just as a ship uses a compass to reach its destination, we use the order of operations to find the correct solution to mathematical problems.
Understanding the Order of Operations – Explanation
The Order of Operations is the agreed-upon standard that dictates the correct sequence for calculating expressions, avoiding ambiguity and ensuring consistency across all mathematical calculations.
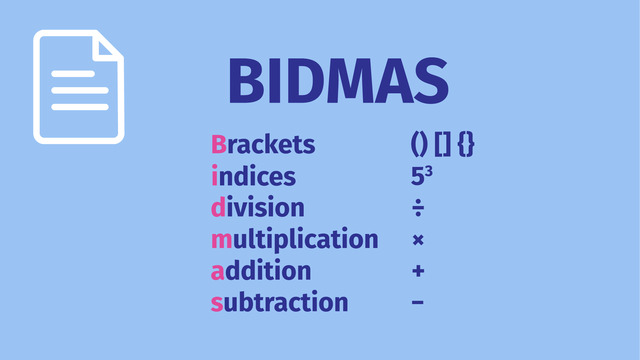
Exploring mathematics involves understanding essential concepts such as the order of operations. We often liken this to navigating through complex pathways, where each step must follow a specific sequence to reach the correct solution. Let's consider the acronym BIDMAS to simplify the Order of Operations:
| Step | Operation Type | Description |
|---|---|---|
| 1 | Brackets (B) | Complete all operations inside bracket symbols first. |
| 2 | Indices (I) | Calculate powers and roots next. |
| 3 | Divide/Multiply (DM) | Perform multiplication and division, from left to right. |
| 4 | Add/Subtract (AS) | Finally, handle subtraction and addition, from left to right. |
Order of Operations – Example
Let's solve an example problem to demonstrate the Order of Operations using the BIDMAS method.
Calculate: $5 + 2 \times (3^{2} - 1)$
Here's how it breaks down using a table:
| Step | Description | Calculation |
|---|---|---|
| Bracket Symbols | Solve the expression within the brackets first. | $3^{2} - 1$ becomes $9 - 1 = 8$ |
| Indices | There are no more indices to calculate after the first. | - |
| Divide/Multiply | Carry out the multiplication next. | $2 \times 8 = 16$ |
| Add/Subtract | Finally, perform the addition. | $5 + 16 = 21$ |
So, $5 + 2 \times (3^{2} - 1) = 21$.
Order of Operations – Guided Practice
Now, let's solve another problem step-by-step:
Calculate: $6 \times (2 + 4) \div 3^{2}$
Try solving the following expression by applying the BIDMAS Order of Operations:
Calculate: $4^{2} - 3 \times (8 \div 2 + 5)$
Order of Operations – Summary
Key Learnings from this Text:
- The Order of Operations is crucial for solving maths problems correctly.
- BIDMAS stands for Brackets, Indices (like powers and roots), Division, Multiplication, Addition and Subtraction.
- Operations inside bracket symbols always come first, followed by exponents (or indices).
- Multiplication and division are performed from left to right, as are subtraction and addition.
- Following this order ensures that everyone arrives at the same correct answer.
To strengthen your understanding and become proficient at maths problems, explore our interactive practice problems, videos and printable worksheets! Have a look at Writing Numerical Expressions next!
Order of Operations – Frequently Asked Questions
