Comparing Decimal Tenth and Hundredth

Basics on the topic Comparing Decimal Tenth and Hundredth
Comparing Decimals – Tenth and Hundredth
What is comparing decimals? When comparing decimals tenths and hundredths, use the greater than, less than or equal to symbols.
In this text on comparing decimals, we practise comparing decimals with models in addition to looking at place value, because base ten blocks help us visualise numbers. Let’s take a look at a comparing decimals example.
Revision – Tenths and Hundredths
Remember, when we talk about decimals, we talk about numbers smaller than 1 (0.86, 0.65, …). The tenth place in a decimal number is the first number after the decimal point. The hundredth place in a decimal number is the second number after the decimal point. Let’s look at an example – 0.57 – in a place value chart.
| Ones | Decimal point | Tenths | Hundredths |
|---|---|---|---|
| 0 | . | 5 | 7 |
Comparing Decimals – Example
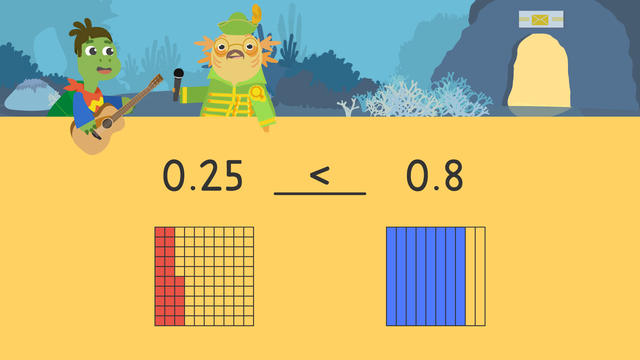
The first labels they have to compare are twenty-five hundredths and eight tenths. Below, we have twenty-five out of one hundred squares shaded in and eight out of ten strips shaded in.
When comparing decimals you need to start with the greatest place value, the ones place. Since the zeros are equal, we move to the next place value, the tenths place. Twenty-five hundredths has a two in the tenths place and eight tenths has an eight in the tenths place.
Since we found a digit greater or less than, we can stop comparing! Twenty-five hundredths is less than eight tenths.
Comparing Decimals – Summary
Remember, when comparing decimals, keep these steps in mind:
| Step # | What to do |
|---|---|
| 1 | Start with the greatest place value and compare. |
| 2 | If the digits are equal, move on to the next place value. |
| 3 | Repeat the process until you find a digit that is greater than or less than and compare using the greater than or the less than symbol. |
| 4 | If the digits are the same and the value shaded in is equal, that means the decimal numbers are equal. |
| 5 | Compare using the equal to symbol. |
- Start with the greatest place value and compare
- If the digits are equal, move on to the next place value
- Repeat the process until you find a digit that is greater than or less than and compare using the greater than or less than symbol
- Or, if the digits are the same and the value shaded in is equal, that means the decimal numbers are equal
- Don't forget to compare using the equal to symbol.
Have you practised yet? On this website you can find more interactive exercises, worksheets and more activities on comparing decimals to the tenths and hundredths.
Transcript Comparing Decimal Tenth and Hundredth
"Whew! Our first day on the job is going swimmingly!" "Turtally! Except, our day just got started." "Erm, right. Look! We have to compare the numbers on the labels to sort the packages into tubs." "The equal packages go in this tub, the smaller value packages go in this tub and the larger value packages go in this tub!" Let's help Axel and Tank by comparing decimals: tenths and hundredths. A decimal number represents an equal part of a whole, just like a fraction. To compare decimals, use the greater than, less than or equal to symbols. The first labels they have to compare are zero point two five and zero point eight. Base ten blocks help us visualise numbers, so let's use them to compare the decimals. Here, we have twenty-five out of one hundred squares shaded in and here, we have eight out of ten strips shaded in. Now, compare starting with the greatest place value, the ones place. Since the zeros are equal, we move to the next place value, the tenths place. Zero point two five has a two in the tenths place and zero point eight has an eight in the tenths place. Since we found a digit greater or less than, we can stop comparing! Zero point two five is less than zero point eight. Next, Axel and Tank need to compare zero point six and zero point six zero. Let's represent them using base ten blocks. Here, we have six out of ten strips shaded in and here, we have sixty out of one hundred squares shaded in. Now, start by comparing the greatest place value, the ones place. Since the zeros are equal, move to the next place value, the tenths place. Both numbers are equal, so we move to the hundredths place. Zero point six doesn't have a digit in the hundredths place, so write a zero as a placeholder. What do you notice about the hundredths place? There are two zeros, making these decimals equal because all of the place value digits are the same. How is that possible? Even though the wholes are broken into different parts, both values are equal! Finally, Axel and Tank need to compare one point two nine and one point two. Let's represent them using base ten blocks. Here, we have one whole and twenty-nine out of one hundred squares shaded in and here, we have one whole and two out of ten strips shaded in. What is the first step? Start by comparing the greatest place value, the ones place. Since the ones are equal, we move to the tenths place. What do you notice about the tenths place? Both numbers are equal, so we move to the hundredths place. One point two doesn't have a digit in the hundredths place, so write a zero as a placeholder. What do you notice about the hundredths place? One point two nine has a nine in the hundredths place and one point two has a zero in the hundredths place so one point two nine is greater than one point two. It looks like one more package came down the belt, and it has Axel and Tank's names on it! Before we see what's inside let's summarise. Remember, when comparing decimals start with the greatest place value, and compare. If the digits are equal, move on to the next place value. Repeat the process until you find a digit that is greater than or less than and compare using the greater than or less than symbol. Or, if the digits are the same and the value shaded in is equal, that means the decimal numbers are equal. Don't forget to compare using the equal to symbol. "I can't believe we got these groovy costumes!" "Yeah! Let's go and explore beneath the waves!"
Comparing Decimal Tenth and Hundredth exercise
-
Where do we start comparing?
HintsWe read numbers from left to right. Which place value do we look at first?
Ones are greater than tenths, and tenths are greater than hundredths.
SolutionWe start comparing in the greatest place value.
When comparing the decimals 0.25 and 0.8 as shown in the model, we start in the ones place because that is the greatest place. Since both decimals have a 0 in the ones place, we would then move on to the next place, the tenths place to compare. Here we can see that 8 is greater than 2, therefore 0.25 < 0.8.
-
Can you match the base ten models and decimals?
HintsLook at the number in the tenths column. There should be that many full rows shaded in.
For example, 0.76 has a 7 in the tenths column so there are 7 full rows shaded plus 6 more individual squares.
A model that is entirely shaded in, represents one whole.
Remember, a decimal is part of a whole.
Solution- 0.27 is represented by a model cut into 100 squares. Since the 2 is in the tenths place, 2 full rows need to be shaded in. Since the 7 is in the hundredths place, 7 individual squares need to be shaded in.
- 0.8 is represented by a model in 10 rows. Since there is an 8 in the tenths place, 8 rows need to be shaded in.
- 1.15 is represented by two models cut into 100 pieces. Since the 1 is in the ones place, one whole model needs to be shaded in. 0.15 is represented by shading in one full row or one tenth, and 5 individual squares for the 5 in the hundredths place.
- 0.12 is represented by a model cut into 100 pieces. One full row is shaded in to represent 1 tenth. Two individual squares are shaded in to show the 2 in the hundredths place.
-
Which decimals are greater than 0.74?
HintsGreater than means bigger or larger.
Start to compare in the greatest place value.
For example, 0.25 and 0.8 both have 0 in the ones place so we move onto the tenths place where we can see that 2 < 8, therefore 0.25 < 0.8.
Grab some paper and a pencil and draw base ten models of each decimal and compare it to the model for 0.74.
If the decimals don't go to the same place, add a 0 as a place holder.
0.60 goes to the hundredths place and 0.6 goes to the tenths place. We can add a 0 in the hundredths place to make the decimal 0.60. You then see that these are equal.
SolutionIn the image above, we can see that 0.8 is greater than 0.74. This is because when you compare the tenths place, 8 is larger than 7. In the model you see 8 rows fully shaded compared to 7 rows fully shaded.
_______________________________________________________
The decimals greater than 0.74 are:
- 0.8
- 0.77
- 1.74
- 0.97
- 0.64 is less than 0.74
- 0.74 is equal to 0.74
-
Which is the correct symbol?
HintsStart comparing by looking at the digit in the greatest place value.
Remember, all decimals must go to the same place. Use zero as a place holder if they do not go to the same place.
For example, 1.54 < 1.65.
Compare the tenths place which are full rows shaded in. The model on the left has five full rows shaded in and the model on the right has six full rows shaded in. Five is less than six.
SolutionThe correct answers are:
- 0.63 > 0.51 because 6 tenths is more than 5 tenths.
- 1.4 = 1.40 because if you add a zero to the hundredths place in 1.4, you see that the decimals are the same.
- 1.07 < 1.70 because 0 tenths is less than than 7 tenths.
- 0.80 > 0.08 because 8 tenths is more than 0 tenths.
- 0.76 < 0.9 because 7 tenths is less than 9 tenths.
-
Compare the decimals using models.
HintsStart with the greatest place value and compare. If the digits are the same, move on until the digits are different.
For example:
- Start by comparing the ones place.
- They have the same digit, so we move to the tenths place.
- They also have the same digit so we move to the hundredths place.
- 1.2 doesn't have a digit in the hundredths place, so we add a 0 as a place holder and then compare.
- 9 hundredths is greater than 0 hundredths, so 1.29 > 1.2.
Remember, when decimals don't go to the same place, add a 0 as a place holder and then compare.
Solution- 0.8 > 0.6; 8 tenths is greater than 6 tenths.
- 0.6 = 0.60; these decimals are equal because when you add a zero to 0.6 it becomes 0.60. Therefore 0.6 and 0.60 are the same.
- 0.1 < 0.4; 1 tenth is less than 4 tenths.
- 0.68 > 0.32; comparing the digits in the tenths place, we can see that 6 > 3 therefore 0.68 > 0.32.
-
Greater than, less than, or equal to 1.2?
HintsRemember, you are comparing each decimal to 1.2. Look at the decimal and compare the place values to 1.2. Draw models to help you if necessary.
Start with the greatest place when comparing.
If the digits are the same, move on to the next digit to compare.
Use a placeholder if necessary.
Solution- 1.24 and 1.4 are greater than 1.2 so you assign them to the > symbol.
- 1.02 and 0.24 are less than 1.2 so you assign them to the < symbol.
- 1.2 and 1.20 are equal to 1.2 so you assign them to the = symbol.
 Do you want to learn faster and more easily?
Do you want to learn faster and more easily?