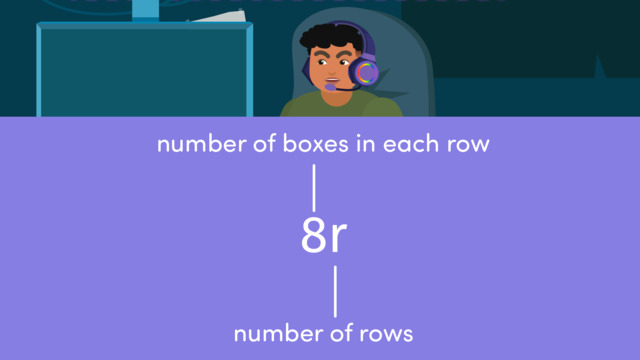
How to Write Expressions
- How to Write Expressions
- How to Write Expressions – Definition
- How to Write Expressions – Step-by-Step Process
- How to Write Expressions – Real-World Application
- How to Write Expressions – Guided Practice
- How to Write Expressions – Practice
- How to Write Expressions – Summary
- How to Write Expressions – Frequently Asked Questions
Learning text on the topic How to Write Expressions
How to Write Expressions
Writing mathematical expressions is a fundamental skill that helps translate real-world situations into manageable mathematical problems. It involves identifying key quantities and their relationships and then using symbols and numbers to express these relationships succinctly. This guide will explore the basics of constructing mathematical expressions, equipping you with the tools to solve problems efficiently and accurately. These expressions use variables so have a look at this text if you’d like a refresher.
How to Write Expressions – Definition
An algebraic expression is a mathematical phrase containing numbers, variables, and operation symbols. In business planning, algebraic expressions can model financial scenarios, calculate costs and predict growth.
Here's a table summarising common keywords associated with each of the basic mathematical operations, which can help in identifying what operation to use when writing mathematical expressions:
| Operation | Keywords |
|---|---|
| Addition | add, plus, increase, total, sum, together, more than |
| Subtraction | subtract, minus, less, decrease, fewer, less than |
| Multiplication | multiply, times, product, doubled, tripled |
| Division | divide, divided by, quotient, per, ratio |
How to Write Expressions – Step-by-Step Process
Here's a concise step-by-step guide on how to write an algebraic expression, followed by two simple examples to demonstrate the process:
Step-by-Step Process of Writing an Algebraic Expression
| Step | Description |
|---|---|
| Step 1: Identify the Variables | Determine what the unknowns are in the problem and assign a variable (like $ x $, $ y $, etc.) to each. |
| Step 2: Determine the Operation | Based on the keywords or problem context, decide which mathematical operations (addition, subtraction, multiplication, division) are required. |
| Step 3: Formulate the Expression | Combine the variables and numbers using the operations identified to create an algebraic expression that represents the problem. |
Let’s look at some examples.
Example 1:: Suppose you have 5 counters and you receive $ x $ additional counters from a friend.
- Identify the Variables: Let $ x $ represent the number of counters received.
- Determine the Operation: The problem involves adding the counters you received to the ones you already have.
- Formulate the Expression: The algebraic expression for this situation is $ 5 + x $.
Example 2:: You buy $ y $ pencils, and each pencil costs 3 dollars.
- Identify the Variables: Let $ y $ represent the number of pencils.
- Determine the Operation: Since each pencil costs a certain amount, you multiply the number of pencils by the cost per pencil.
- Formulate the Expression: The algebraic expression for this situation is $ 3y $.
How to Write Expressions – Real-World Application
Example: For Mateo's taco catering business, he has a simple pricing model. He sells each taco for $£2.50$ and charges an additional service fee of $£200$. An algebraic expression to represent Mateo's total charge for 't' tacos is:
$2.50t + 200$
With '$t$' representing the number of tacos sold, we can calculate Mateo's revenue by substituting the value of '$t$' into the expression.
Mateo serves three tacos per person and needs to account for kitchen accidents that usually cost him 10 tacos. To calculate the number of people Mateo can serve, we use the following expression:
$(t - 10) \div 3$
This expression takes the total number of tacos '$t$', subtracts the $10$ accident-prone tacos, and divides by $3$ since each person gets three tacos.
Mateo projects that his company's value will double each week. To model this exponential growth, we use the following expression:
$10,000 × 2^{w}$
Here, 'w' represents the number of weeks, and the entire expression predicts the company's value after 'w' weeks.
How to Write Expressions – Guided Practice
1. Hannah makes and sells handmade necklaces. She charges $£15$ for each necklace and a fixed shipping fee of $£5$ per order.
Algebraic Expression: The total charge for 'n' necklaces would be: $ 15n + 5 $
With '$n$' representing the number of necklaces sold, we can calculate Hannah's revenue by substituting the value of '$n$' into the expression.
2. Alex tutors students and charges $£20$ per hour. Additionally, he charges a one-time material fee of $£10$ per student.
Algebraic Expression: The total charge for 'h' hours of tutoring would be: $ 20h + 10 $
With '$h$' representing the number of tutoring hours, we can calculate Alex's total earnings by substituting the value of '$h$' into the expression.
How to Write Expressions – Practice
How to Write Expressions – Summary
Key Learnings from this Text:
- Algebraic expressions can simplify financial calculations in business planning.
- Translating business scenarios into algebraic expressions is essential for strategic planning and forecasting.
Engage further in exploration of the interplay between algebra and real-world applications like business planning, through our engaging content, interactive practice problems, and additional learning resources on our platform.
 Do you want to learn faster and more easily?
Do you want to learn faster and more easily?